Essentials of Corporate Finance 9 None

Solutions Manual

Essentials of Corporate Finance

Ross, Westerfield, and Jordan

9th edition

01/03/2016

Prepared by

Brad Jordan

University of Kentucky

Joe Smolira

Belmont University

CHAPTER 1

INTRODUCTION TO CORPORATE

FINANCE

Answers to Concepts Review and Critical Thinking Questions

6. In the corporate form of ownership, the shareholders are the owners of the firm. The shareholders elect

CHAPTER 1 – 2

10. An argument can be made either way. At one extreme, we could argue that in a market economy, all

of these things are priced. This implies an optimal level of ethical and/or illegal behavior and the

framework of stock valuation explicitly includes these. At the other extreme, we could argue that these

are non-economic phenomena and are best handled through the political process. The following is a

classic (and highly relevant) thought question that illustrates this debate: “A firm has estimated that

the cost of improving the safety of one of its products is $30 million. However, the firm believes that

improving the safety of the product will only save $20 million in product liability claims. What should

the firm do?”

12. The goal of management should be to maximize the share price for the current shareholders. If

management believes that it can improve the profitability of the firm so that the share price will exceed

13. We would expect agency problems to be less severe in other countries, primarily due to the relatively

small percentage of individual ownership. Fewer individual owners should reduce the number of

14. How much is too much? Who is worth more, Michael Fries or LeBron James? The simplest answer is

that there is a market for executives just as there is for all types of labor. Executive compensation is

the price that clears the market. The same is true for athletes and performers. Having said that, one

3 – SOLUTIONS MANUAL

15. The biggest reason that a company would “go dark” is because of the increased audit costs associated

with Sarbanes-Oxley compliance. A company should always do a cost-benefit analysis, and it may be

the case that the costs of complying with Sarbox outweigh the benefits. Of course, the company could

always be trying to hide financial issues of the company! This is also one of the costs of going dark:

Investors surely believe that some companies are going dark to avoid the increased scrutiny from

CHAPTER 2

WORKING WITH FINANCIAL

STATEMENTS

Answers to Concepts Review and Critical Thinking Questions

5. Market values can never be negative. Imagine a share of stock selling for –$20. This would mean that

if you placed an order for 100 shares, you would get the stock along with a check for $2,000. How

8. For example, if a company were to become more efficient in inventory management, the amount of

5 – SOLUTIONS MANUAL

10. The adjustments discussed were purely accounting changes; they had no cash flow or market value

consequences unless the new accounting information caused stockholders to revalue the company.

Solutions to Questions and Problems

1. The balance sheet for the company will look like this:

Balance sheet

Current assets

$2,030

Current liabilities

$1,640

Net fixed assets

9,780

Long-term debt

4,490

Owners’ equity

5,680

Total assets

$11,810

Total liabilities and owners’ equity

$11,810

CHAPTER 2 – 6

2. The income statement starts with revenues and subtracts costs to arrive at EBIT. We then subtract out

interest to get taxable income, and then subtract taxes to arrive at net income. Doing so, we get:

Income Statement

4. Earnings per share is the net income divided by the shares outstanding, so:

EPS = Net income / Shares outstanding

6. The average tax rate is the total taxes paid divided by taxable income, so:

Average tax rate = Total tax / Taxable income

7 – SOLUTIONS MANUAL

7. To calculate the OCF, we first need to construct an income statement. The income statement starts

with revenues and subtracts costs to arrive at EBIT. We then subtract out interest to get taxable income,

and then subtract taxes to arrive at net income. Doing so, we get:

Income Statement

Sales $38,530

Costs 12,750

9. The change in net working capital is the end of period net working capital minus the beginning of

period net working capital, so:

10. The cash flow to creditors is the interest paid, minus any net new borrowing, so:

11. The cash flow to stockholders is the dividends paid minus any new equity raised. So, the cash flow to

stockholders is: (Note that APIS is the additional paid-in surplus.)

12. We know that cash flow from assets is equal to cash flow to creditors plus cash flow to stockholders.

So, cash flow from assets is:

CHAPTER 2 – 8

Cash flow from assets = Cash flow to creditors + Cash flow to stockholders

Cash flow from assets = –$38,200 – 155,500

Cash flow from assets = –$193,700

13. To find the book value of current assets, we use: NWC = CA – CL. Rearranging to solve for current

assets, we get:

14. a. To calculate the OCF, we first need to construct an income statement. The income statement starts

with revenues and subtracts costs to arrive at EBIT. We then subtract out interest to get taxable

income, and then subtract taxes to arrive at net income. Doing so, we get:

Income Statement

Sales $173,000

Costs 91,400

Other Expenses 5,100

Depreciation 12,100

EBIT $64,400

Interest 8,900

Taxable income $55,500

Taxes 21,090

Net income $34,410

Dividends $9,700

Addition to retained earnings 24,710

9 – SOLUTIONS MANUAL

Dividends paid plus addition to retained earnings must equal net income, so:

Net income = Dividends + Addition to retained earnings

Addition to retained earnings = $34,410 – 9,700

Addition to retained earnings = $24,710

So, the operating cash flow is:

OCF = EBIT + Depreciation – Taxes

OCF = $64,400 + 12,100 – 21,090

OCF = $55,410

CHAPTER 2 – 10

15. Here we need to work the income statement backward. Starting with net income, we know that net

income is:

Net income = Dividends + Addition to retained earnings

Net income = $2,170 + 3,500

Net income = $5,670

Net income is also the taxable income, minus the taxable income times the tax rate, or:

Net income = Taxable income – (Taxable income)(Tax rate)

Net income = Taxable income(1 – Tax rate)

We can rearrange this equation and solve for the taxable income as:

16. We can fill in the balance sheet with the numbers we are given. The balance sheet will be:

Balance Sheet

Cash $197,000 Accounts payable $288,000

Accounts receivable 265,000 Notes payable 194,000

Inventory 563,000 Current liabilities $482,000

Current assets $1,025,000 Long-term debt 1,490,000

Total liabilities $2,072,000

Tangible net fixed assets $5,150,000

11 – SOLUTIONS MANUAL

17. Owners’ equity is the maximum of total assets minus total liabilities, or zero. Although the book value

of owners’ equity can be negative, the market value of owners’ equity cannot be negative, so:

18. a. Using Table 2.3, we can see the marginal tax schedule. For Corporation Growth, the first $50,000

of income is taxed at 15 percent, the next $25,000 is taxed at 25 percent, and the next $1,500 is

taxed at 34 percent. So, the total taxes for the company will be:

TaxesGrowth = .15($50,000) + .25($25,000) + .34($1,500)

TaxesGrowth = $14,260

CHAPTER 2 – 12

19. a. The income statement starts with revenues and subtracts costs to arrive at EBIT. We then subtract

interest to get taxable income, and then subtract taxes to arrive at net income. Doing so, we get:

Income Statement

Sales $2,350,000

Cost of goods sold 1,925,000

Admin expenses 530,000

Depreciation 420,000

EBIT $ 105,000

20. A firm can still pay out dividends if net income is negative; it just has to be sure there is sufficient

cash flow to make the dividend payments. The assumptions made in the question are:

Change in NWC = Net capital spending = Net new equity = 0

To find the new long-term debt, we first need to find the cash flow from assets. The cash flow from

assets is:

Cash flow from assets = OCF – Change in NWC – Net capital spending

Cash flow from assets = $525,000 – 0 – 0

Cash flow from assets = $525,000

We can also find the cash flow to stockholders, which is:

13 – SOLUTIONS MANUAL

21. a. To calculate the OCF, we first need to construct an income statement. The income statement starts

with revenues and subtracts costs to arrive at EBIT. We then subtract out interest to get taxable

income, and then subtract taxes to arrive at net income. Doing so, we get:

Income Statement

Sales $28,476

Cost of goods sold 20,136

Depreciation 3,408

EBIT $ 4,932

Interest 497

Taxable income $ 4,435

Taxes (40%) 1,774

Net income $ 2,661

b. The operating cash flow for the year was:

OCF = EBIT + Depreciation – Taxes

OCF = $4,932 + 3,408 – 1,774

OCF = $6,566

c. To calculate the cash flow from assets, we also need the change in net working capital and net

capital spending. The change in net working capital was:

Change in NWC = NWCend – NWCbeg

Change in NWC = (CAend – CLend) – (CAbeg – CLbeg)

Change in NWC = ($4,234 – 2,981) – ($3,528 – 3,110)

Change in NWC = $835

And the net capital spending was:

Net capital spending = NFAend – NFAbeg + Depreciation

CHAPTER 2 – 14

22. a. To calculate owners’ equity, we first need total liabilities and owners’ equity. From the balance

sheet relationship we know that this is equal to total assets. We are given the necessary information

to calculate total assets. Total assets are current assets plus fixed assets, so:

Total assets = Current assets + Fixed assets = Total liabilities and owners’ equity

For 2015, we get:

Total assets = $2,718 + 12,602

Total assets = $15,320

Now, we can solve for owners’ equity as:

Total liabilities and owners’ equity = Current liabilities + Long-term debt + Owners’ equity

$15,320 = $1,174 + 6,873 + Owners’ equity

Owners’ equity = $7,273

For 2016, we get:

Total assets = $2,881 + 13,175

Total assets = $16,056

15 – SOLUTIONS MANUAL

Now we can solve for owners’ equity as:

Total liabilities and owners’ equity = Current liabilities + Long-term debt + Owners’ equity

$16,056 = $1,726 + 8,019 + Owners’ equity

Owners’ equity = $6,311

b. The change in net working capital was:

Change in NWC = NWCend – NWCbeg

Change in NWC = (CAend – CLend) – (CAbeg – CLbeg)

Change in NWC = ($2,881 – 1,726) – ($2,718 – 1,174)

Change in NWC = –$389

c. To find the amount of fixed assets the company sold, we need to find the net capital spending. The

net capital spending was:

Net capital spending = NFAend – NFAbeg + Depreciation

Net capital spending = $13,175 – 12,602 + 3,434

Net capital spending = $4,007

To find the fixed assets sold, we can also calculate net capital spending as:

Net capital spending = Fixed assets bought – Fixed assets sold

$4,007 = $7,160 – Fixed assets sold

Fixed assets sold = $3,153

CHAPTER 2 – 16

23. To construct the cash flow identity, we will begin with cash flow from assets. Cash flow from assets

is:

Cash flow from assets = OCF – Change in NWC – Net capital spending

So, the operating cash flow is:

OCF = EBIT + Depreciation – Taxes

OCF = $103,562 + 69,038 – 27,703

OCF = $144,897

Next, we will calculate the change in net working capital, which is:

Change in NWC = NWCend – NWCbeg

Change in NWC = (CAend – CLend) – (CAbeg – CLbeg)

Change in NWC = ($73,571 – 34,127) – ($58,325 – 30,352)

Change in NWC = $11,471

Now, we can calculate the capital spending. The capital spending is:

Net capital spending = NFAend – NFAbeg + Depreciation

Net capital spending = $513,980 – 435,670 + 69,038

Net capital spending = $147,348

Now, we have the cash flow from assets, which is:

Cash flow from assets = OCF – Change in NWC – Net capital spending

Cash flow from assets = $144,897 – 11,471 – 147,348

Cash flow from assets = –$13,922

17 – SOLUTIONS MANUAL

25. a. The tax bubble causes average tax rates to catch up to marginal tax rates, thus eliminating the tax

advantage of low marginal rates for high-income corporations.

CHAPTER 2 – 18

b. Taxes = .15($50K) + .25($25K) + .34($25K) + .39($235K) = $113.9K

Average tax rate = $113.9K / $335K = 34%

The marginal tax rate on the next dollar of income is 34 percent.

For corporate taxable income levels of $335K to $10M, average tax rates are equal to marginal tax

rates.

CHAPTER 3

WORKING WITH FINANCIAL

STATEMENTS

Answers to Concepts Review and Critical Thinking Questions

1. a. If inventory is purchased with cash, then there is no change in the current ratio. If inventory is

purchased on credit, then there is a decrease in the current ratio if it was initially greater than 1.0.

b. Reducing accounts payable with cash increases the current ratio if it was initially greater than 1.0.

c. Reducing short-term debt with cash increases the current ratio if it was initially greater than 1.0.

4. a. Quick ratio provides a measure of the short-term liquidity of the firm, after removing the effects

of inventory, generally the least liquid of the firm’s current assets.

b. Cash ratio represents the ability of the firm to completely pay off its current liabilities balance with

its most liquid asset (cash).

c. The capital intensity ratio tells us the dollar amount investment in assets needed to generate one

dollar in sales.

20 – SOLUTIONS

6. Peer group analysis involves comparing the financial ratios and operating performance of a particular

firm to a set of peer group firms in the same industry or line of business. Comparing a firm to its peers

7. Return on equity is probably the most important accounting ratio that measures the bottom-line

CHAPTER 3 – 21

10. a. For an electric utility such as Con Ed, expressing costs on a per kilowatt-hour basis would be a

way of comparing costs with other utilities of different sizes.

b. For a retailer such as JC Penney, expressing sales on a per square foot basis would be useful in

comparing revenue production against other retailers.

11. As with any ratio analysis, the ratios themselves do not necessarily indicate a problem, but simply

indicate that something is different and it is up to us to determine if a problem exists. If the cost of

goods sold as a percentage of sales is increasing, we would expect that EBIT as a percentage of sales

would decrease, all else constant. An increase in the cost of goods sold as a percentage of sales occurs

12. If we assume that the cause is negative, the two reasons for the trend of increasing cost of goods sold

as a percentage of sales are that costs are becoming too high or the sales price is not increasing fast

enough. If the cause is an increase in the cost of goods sold, the manager should look at possible

actions to control costs. If costs can be lowered by seeking lower cost suppliers of similar or higher

quality, the cost of goods sold as a percentage of sales should decrease. Another alternative is to

increase the sales price to cover the increase in the cost of goods sold. Depending on the industry, this

22 – SOLUTIONS

1. To find the current assets, we must use the net working capital equation. Doing so, we find:

NWC = Current assets – Current liabilities

$1,965 = Current assets – $5,460

Current assets = $7,425

Now, use this number to calculate the current ratio and the quick ratio. The current ratio is:

2. To find the return on assets and return on equity, we need net income. We can calculate the net income

using the profit margin. Doing so, we find the net income is:

Profit margin = Net income / Sales

.07 = Net income / $13,500,000

Net income = $945,000

Now we can calculate the return on assets as:

CHAPTER 3 – 23

3. The receivables turnover for the company was:

Receivables turnover = Credit sales / Receivables

4. The inventory turnover for the company was:

Inventory turnover = COGS / Inventory

5. To find the debt–equity ratio using the total debt ratio, we need to rearrange the total debt ratio

equation. We must realize that the total assets are equal to total debt plus total equity. Doing so, we

find:

Total debt ratio = Total debt / Total assets

24 – SOLUTIONS

6. We need to calculate the net income before we calculate the earnings per share. The sum of dividends

and addition to retained earnings must equal net income, so net income must have been:

Net income = Addition to retained earnings + Dividends

Net income = $534,000 + 185,000

Net income = $719,000

So, the earnings per share were:

EPS = Net income / Shares outstanding

EPS = $719,000 / 365,000

EPS = $1.97 per share

The dividends per share were:

Dividends per share = Total dividends / Shares outstanding

Dividends per share = $185,000 / 365,000

Dividends per share = $.51 per share

The book value per share was:

Book value per share = Total equity / Shares outstanding

CHAPTER 3 – 25

8. We can use the Du Pont identity and solve for the equity multiplier. With the equity multiplier we can

9. To find the days’ sales in payables, we first need to find the payables turnover. The payables turnover

was:

Payables turnover = Cost of goods sold / Payables balance

Payables turnover = $87,386 / $19,472

Payables turnover = 4.49 times

10. With the information provided, we need to calculate the return on equity using an extended return on

equity equation. We first need to find the equity multiplier, which is:

Equity multiplier = 1 + Debt–equity ratio

Equity multiplier = 1 + .75

Equity multiplier = 1.75

26 – SOLUTIONS

Now we can calculate the return on equity as:

11. To find the internal growth rate, we need the plowback, or retention, ratio. The plowback ratio is:

b = 1 – .25

12. To find the sustainable growth rate we need the plowback, or retention, ratio. The plowback ratio is:

b = 1 – .20

13. We need the return on equity to calculate the sustainable growth rate. To calculate return on equity,

we need to realize that the total asset turnover is the inverse of the capital intensity ratio and the equity

multiplier is one plus the debt–equity ratio. So, the return on equity is:

ROE = (Profit margin)(Total asset turnover)(Equity multiplier)

ROE = (.081)(1/.45)(1 + .55)

ROE = .2790, or 27.90%

14. We need the return on equity to calculate the sustainable growth rate. Using the Du Pont identity, the

return on equity is:

ROE = (Profit margin)(Total asset turnover)(Equity multiplier)

ROE = (.057)(2.80)(1.47)

15. To calculate the common-size balance sheet, we divide each asset account by total assets, and each

liability and equity account by total liabilities and equity. For example, the common-size cash

percentage for 2015 is:

28 – SOLUTIONS

Net plant and equipment

$705,386

80.10%

$785,205

79.24%

16. a. The current ratio is calculated as:

Curent ratio = Current assets / Current liabilities

Current ratio2015 = $175,278 / $250,749

Current ratio2015 = .70 times

Current ratio2016 = $205,685 / $261,590

Current ratio2016 = .79 times

CHAPTER 3 – 29

b. The quick ratio is calculated as:

Quick ratio = (Current assets – Inventory) / Current liabilities

Quick ratio2015 = ($175,278 – 109,626) / $250,749

Quick ratio2015 = .26 times

Quick ratio2016 = ($205,685 – 129,253) / $261,590

Quick ratio2016 = .29 times

c. The cash ratio is calculated as:

Cash ratio = Cash / Current liabilities

Cash ratio2015 = $19,256 / $250,749

Cash ratio2015 = .08 times

Cash ratio2016 = $21,946 / $261,590

Cash ratio2016 = .08 times

30 – SOLUTIONS

17. Using the Du Pont identity to calculate ROE, we get:

18. One equation to calculate ROA is:

ROA = (Profit margin)(Total asset turnover)

We can solve this equation to find total asset turnover as:

19. To calculate the ROA, we first need to find the net income. Using the profit margin equation, we find:

Profit margin = Net income / Sales

20. To calculate the internal growth rate, we need to find the ROA and the plowback ratio. The ROA for

the company is:

ROA = Net income / Total assets

ROA = $15,112 / $120,400

ROA = .1255, or 12.55%

21. To calculate the sustainable growth rate, we need to find the ROE and the plowback ratio. The ROE

for the company is:

ROE = Net income / Equity

22. The total asset turnover is:

23. To find the ROE, we need the equity balance. Since we have the total debt, if we can find the total

assets we can calculate the equity. Using the total debt ratio, we find total assets as:

Debt ratio = Total debt / Total assets

.75 = $353,000 / Total assets

Total assets = $470,667

32 – SOLUTIONS

24. The earnings per share are:

PE = 31.76 times

The sales per share are:

Sales per share = Sales / Shares

Sales per share = $52,800,000 / 4,600,000

Sales per share = $11.48

The price–sales ratio is:

P/S = Price / Sales per share

CHAPTER 3 – 33

1.80 = Sales / $7,450,000

Sales = $13,410,000

And the net income is:

26. First, we need the enterprise value, which is:

Enterprise value = Market capitalization + Debt – Cash

Enterprise value = $635,000 + 215,000 – 39,000

Enterprise value = $811,000

And EBITDA is:

27. We can rearrange the Du Pont identity to calculate the profit margin. So, we need the equity multiplier

and the total asset turnover. The equity multiplier is:

Equity multiplier = 1 + Debt–equity ratio

Equity multiplier = 1 + .25

Equity multiplier = 1.25

34 – SOLUTIONS

And the total asset turnover is:

1.30 = Current assets / $2,435

Current assets = $3,165.50

Now, we are going to use the profit margin to find the net income and use the net income to find the

equity. Doing so, we get:

Profit margin = Net income / Sales

.09 = Net income / $11,610

Net income = $1,044.90

And using this net income figure in the return on equity equation to find the equity, we get:

ROE = Net income / Total equity

.128 = $1,044.90 / Total equity

Total equity = $8,163.28

Now, we can use the long-term debt ratio to find the total long-term debt. The equation is:

Long-term debt ratio = Long-term debt / (Long-term debt + Total equity)

CHAPTER 3 – 35

Inverting both sides we get:

29. The child’s profit margin is:

Profit margin = Net income / Sales

Profit margin = $2 / $50

Profit margin = .04, or 4%

And the store’s profit margin is:

36 – SOLUTIONS

12.42 = Sales / $145,350

Sales = $1,805,744

So, the profit margin is:

Profit margin = Net income / Sales

Profit margin = $162,840 / $1,805,744

Profit margin = .0902, or 9.02%

31. Here, we need to work the income statement backward to find the EBIT. Starting at the bottom of the

income statement, we know that the taxes are the taxable income times the tax rate. The net income is

the taxable income minus taxes. Rearranging this equation, we get:

Net income = Taxable income – (TC)(Taxable income)

Net income = (1 – TC)(Taxable income)

Using this relationship we find the taxable income is:

Net income = (1 – TC)(Taxable income)

CHAPTER 3 – 37

32. To find the times interest earned, we need the EBIT and interest expense. EBIT is sales minus costs

minus depreciation, so:

EBIT = Sales – Costs – Depreciation

$1.35 = Dividends / 20,000

Dividends = $27,000

Net income is the sum of dividends and addition to retained earnings, so:

Net income = Dividends + Addition to retained earnings

Net income = $27,000 + 72,800

Net income = $99,800

We know that the taxes are the taxable income times the tax rate. The net income is the taxable income

minus taxes. Rearranging this equation, we get:

38 – SOLUTIONS

33. To find the return on equity, we need the net income and total equity. We can use the total debt ratio

to find the total assets as:

Total debt ratio = Total debt / Total assets

.37 = $673,000 / Total assets

Total assets = $1,818,919

Using the balance sheet relationship that total assets is equal to total liabilities and equity, we find the

total equity is:

34. The currency is generally irrelevant in calculating any financial ratio. The company’s profit margin is:

Profit margin = Net income / Sales

Profit margin = –£27,860 / £512,621

Profit margin = –.0543, or –5.43%

As long as both net income and sales are measured in the same currency, there is no problem; in fact,

CHAPTER 3 – 39

35. Here, we need to calculate several ratios given the financial statements. The ratios are:

Short-term solvency ratios:

Current ratio = Current assets / Current liabilities

Current ratio2015 = $28,666 / $6,319

Current ratio2015 = 4.54 times

Current ratio2016 = $32,409 / $7,427

Current ratio2016 = 4.36 times

Quick ratio = (Current assets – Inventory) / Current liabilities

Quick ratio2015 = ($28,666 – 17,357) / $6,319

Quick ratio2015 = 1.79 times

Quick ratio2016 = ($32,409 – 19,350) / $7,427

Quick ratio2016 = 1.76 times

Cash ratio = Cash / Current liabilities

Cash ratio2015 = $4,607 / $6,319

Cash ratio2015 = .73 times

Cash ratio2016 = $4,910 / $7,427

Cash ratio2016 = .66 times

Asset utilization ratios:

Total asset turnover = Sales / Total assets

Total asset turnover = $205,227 / $109,219

Total asset turnover = 1.88 times

Inventory turnover = COGS / Inventory

Inventory turnover = $138,383 / $19,350

Inventory turnover = 7.15 times

Receivables turnover = Sales / Receivables

Receivables turnover = $205,227 / $8,149

Receivables turnover = 25.18 times

40 – SOLUTIONS

Long-term solvency ratios:

CHAPTER 3 – 41

36. The Du Pont identity is:

37. To find the price–earnings ratio we first need the earnings per share. The earnings per share are:

EPS = Net income / Shares outstanding

EPS = $38,557 / 10,000 shares

EPS = $3.86

So, the price–earnings ratio is:

PE ratio = Share price / EPS

PE ratio = $73 / $3.86

PE ratio = 18.93 times

The sales per share are:

Sales per share = Sales / Shares outstanding

Sales per share = $205,227 / 10,000

Sales per share = $20.52

So, the price–sales ratio is:

42 – SOLUTIONS

38. The current ratio appears to be relatively high when compared to the median; however, it is below the

upper quartile, meaning that at least 25 percent of firms in the industry have a higher current ratio.

Overall, it does not appear that the current ratio is out of line with the industry. The total asset turnover

39. To find the profit margin, we can solve the Du Pont identity. First, we need to find the retention ratio.

The retention ratio for the company is:

b = 1 – .30

b = .70

Now, we can use the sustainable growth rate equation to find the ROE. Doing so, we find:

Sustainable growth rate = [(ROE)(b)] / [1 – (ROE)(b)]

40. The earnings per share is the net income divided by the shares outstanding. Since all numbers are in

millions, the earnings per share for Abercrombie & Fitch was:

EPS = $51.821 / 68.98

EPS = $.75

And the earnings per share for American Eagle Outfitters was:

EPS = $80.322 / 195.43

EPS = $.41

CHAPTER 3 – 43

The market-to-book ratio is the stock price divided by the book value per share. To find the book value

41. To find the total asset turnover, we can solve the ROA equation. First, we need to find the retention

ratio. The retention ratio for the company is:

b = 1 – .25

b = .75

Now, we can use the internal growth rate equation to find the ROA. Doing so, we find:

44 – SOLUTIONS

42. To calculate the sustainable growth rate, we need to calculate the return on equity. We can use the Du

Pont identity to calculate the return on equity if we can find the equity multiplier. Using the total debt

ratio, we can find the debt–equity ratio is:

Total debt ratio = Total debt / Total assets

.30 = Total debt / Total assets

1 / .30 = Total assets / Total debt

1 / .30 = (Total debt + Total equity) / Total debt

1 / .30 = 1 + Total equity / Total debt

Total equity / Total debt = (1 / .30) – 1

Total debt / Total equity = 1 / [(1 /.30) – 1]

Total debt / Total equity = .43

Debt–equity ratio = .43

So, the equity multiplier is:

Equity multiplier = 1 + Debt–equity ratio

CHAPTER 3 – 45

43. To find the sustainable growth rate, we need the retention ratio and the return on equity. The payout

ratio is the dividend payment divided by net income, so:

b = 1 – ($8,100 / $19,000)

b = .5737

And the return on equity is:

ROE = Net income / Total equity

ROE = $19,000 / $91,000

ROE = .2088, or 20.88%

So, the sustainable growth rate is:

Sustainable growth rate = [(ROE)(b)] / [1 – (ROE)(b)]

Sustainable growth rate = [.2088(.5737)] / [1 – .2088(.5737)]

Sustainable growth rate = .1361, or 13.61%

The total assets of the company are equal to the total debt plus the total equity. The total assets will

increase at the sustainable growth rate, so the total assets next year will be:

New total assets = (1 + Sustainable growth rate)(Total assets)

New total assets = (1 + .1361)($67,000 + 91,000)

New total assets = $179,500.62

46 – SOLUTIONS

44. We can find the payout ratio from the sustainable growth rate formula. First, we need the return on

equity. Using the Du Pont identity, we find the return on equity is:

b = 1.29

So, the payout ratio is:

Payout ratio = 1 – b

45. Using the beginning of period total assets, the ROA is:

ROABegin = $1,233 / $14,013

ROABegin = .0880, or 8.80%

Using the end of period total assets, the ROA is:

ROAEnd = $1,233 / $15,256

ROAEnd = .0808, or 8.08%

The ROE using beginning of period equity is:

ROEBegin = $1,233 / $3,986

ROEBegin = .3093, or 30.93%

CHAPTER 3 – 47

The ROE using the end of period equity is:

ROEEnd = $1,233 / $4,995

ROEEnd = .2468, or 24.68%

The retention ratio, which is one minus the dividend payout ratio, is:

b = 1 – Dividends/Net income

b = 1 – $251 / $1,233

b = .7964, or 79.64%

With the growth rate equations, we need to use the ROA and ROE based on the end of period assets

or equity, so the internal growth rate is:

Internal growth rate = [(ROA)(b)] / [1 – (ROA)(b)]

48 – SOLUTIONS

$846.912

$7,421.768

$5,629.516

CHAPTER 4

INTRODUCTION TO VALUATION: THE

TIME VALUE OF MONEY

Answers to Concepts Review and Critical Thinking Questions

8. The key considerations would be: (1) Is the rate of return implicit in the offer attractive relative to

10. The price would be higher because, as time passes, the price of the security will tend to rise toward

$100,000. This rise is just a reflection of the time value of money. As time passes, the time until receipt

of the $100,000 grows shorter, and the present value rises. In 2018, the price will probably be higher

for the same reason. We cannot be sure, however, because interest rates could be much higher, or

TMCC’s financial position could deteriorate. Either event would tend to depress the security’s price.

1. The time line for the cash flows is:

0

10

$8,100

FV

The simple interest per year is:

$8,100 × .06 = $486

So, after 10 years, you will have:

2. To find the FV of a lump sum, we use:

FV = PV(1 + r)t

0

7

$3,150

FV

FV = $3,150(1.13)7 = $7,410.71

0

16

CHAPTER 4 – 51

3. To find the PV of a lump sum, we use:

PV = FV / (1 + r)t

0

15

PV

$17,328

PV = $17,328 / (1.07)15 = $6,280.46

0

8

4. To answer this question, we can use either the FV or the PV formula. Both will give the same answer

since they are the inverse of each other. We will use the FV formula, that is:

FV = PV(1 + r)t

Solving for r, we get:

r = (FV / PV)1 / t – 1

0

11

–$715

$1,381

52 – SOLUTIONS

FV = $1,381 = $715(1 + r)11

r = ($1,381 / $715)1/11 – 1

r = .0617, or 6.17%

0

8

5. To answer this question, we can use either the FV or the PV formula. Both will give the same answer

since they are the inverse of each other. We will use the FV formula, that is:

FV = PV(1 + r)t

t = 17.39 years

CHAPTER 4 – 53

t = 7.51 years

t = 16.95 years

6. The time line is:

0

18

–$53,000

$295,000

7. To find the length of time for money to double, triple, etc., the present value and future value are

irrelevant as long as the future value is twice the present value for doubling, three times as large for

tripling, etc. To answer this question, we can use either the FV or the PV formula. Both will give the

same answer since they are the inverse of each other. We will use the FV formula, that is:

FV = PV(1 + r)t

t = 15.09 years

The length of time to quadruple your money is:

8. The time line is:

0

140

–$20

$15,000

9. The time line is:

0

t

–$35,000

$150,000

CHAPTER 4 – 55

10. The time line is:

0

25

11. The time line is:

0

80

12. The time line is:

0

111

50

FV

56 – SOLUTIONS

13. The time line is:

0

119

$150

$1,620,000

To answer this question, we can use either the FV or the PV formula. Both will give the same answer

since they are the inverse of each other. We will use the FV formula, that is:

FV = PV(1 + r)t

Solving for r, we get:

14. The time line is:

0

76

–$.10

$3,207,852

CHAPTER 4 – 57

15. The time line is:

0

4

–$12,377,500

$10,311,500

16. To answer this question, we can use either the FV or the PV formula. Both will give the same answer

since they are the inverse of each other. We will use the FV formula, that is:

FV = PV(1 + r)t

Solving for r, we get:

r = (FV / PV)1 / t – 1

a. The time line is:

0

20

–$50

$100

58 – SOLUTIONS

–$50.50

$100

17. The time line is:

0

10

18. To find the FV of a lump sum, we use:

FV = PV(1 + r)t

0

45

$5,000

FV

19. The time line is:

0

2

8

$13,000

FV

20. The time line is:

0

2

t

$30,000

$150,000

21. To find the FV of a lump sum, we use:

FV = PV(1 + r)t

In Regency Bank, you will have:

0

240

$6,150

FV

22. The time line is:

0

t

–$1

$3

60 – SOLUTIONS

To find the length of time for money to double, triple, etc., the present value and future value are

23. The time line is:

0

t

–$1,800

$3,100

24. The time line is:

0

120

25. The time line is:

0

45

PV

$1,000,000

To find the PV of a lump sum, we use:

PV = FV / (1 + r)t

26. The time line is:

0

40

–$20,000

FV

In this case, we have an investment that earns two different interest rates. We will calculate the value

of the investment at the end of the first 20 years then use this value with the second interest rate to find

the final value at the end of 40 years. Using the future value equation, at the end of the first 20 years,

the account will be worth:

Value in 20 years = PV(1 + r)t

Value in 20 years = $20,000(1.07)20

Value in 20 years = $77,393.69

1.

62 – SOLUTIONS

2.

Enter

7

13%

$3,150

N

I/Y

PV

PMT

FV

Solve for

$7,410.71

Enter

16

7%

3.

Enter

15

7%

$17,328

N

I/Y

PV

PMT

FV

Solve for

–$6,280.46

Solve for

6.17%

Solve for

8.34%

Solve for

10.26%

Solve for

17.39

Solve for

7.51

Solve for

16.95

64 – SOLUTIONS

Solve for

3.53%

CHAPTER 4 – 65

21.

Enter

240

1%

$6,150

66 – SOLUTIONS

26.

Enter

20

7%

CHAPTER 5

DISCOUNTED CASH FLOW VALUATION

Answers to Concepts Review and Critical Thinking Questions

10. The problem is that the subsidy makes it easier to repay the loan, not obtain it. However, the ability to

repay the loan depends on future employment, not current need. For example, consider a student who

is currently needy, but is preparing for a career in a high-paying area (such as corporate finance!).

Should this student receive the subsidy? How about a student who is currently not needy, but is

preparing for a relatively low-paying job (such as becoming a college professor)?

1. The time line is:

0

1

2

3

4

PV

$680

$490

$975

$1,160

2. The times lines are:

0

1

2

3

4

5

6

7

8

9

PV

$3,400

0

1

2

3

4

5

PV

$5,200

To find the PVA, we use the equation:

PVA = C({1 – [1/(1 + r)t]} / r )

At an interest rate of 6 percent:

CHAPTER 5 – 69

3. The time line is:

0

1

2

3

4

$985

$1,160

$1,325

$1,495

4. To find the PVA, we use the equation:

PVA = C({1 – [1/(1 + r)t]} / r)

0

1

…

15

PV

$5,450

PVA@15 yrs: PVA = $5,450{[1 – (1/1.08)15] / .08} = $46,649.16

0

1

…

40

PV

$5,450

PVA@40 yrs: PVA = $5,450{[1 – (1/1.08)40] / .08} = $64,989.14

0

1

75

70 – SOLUTIONS

5. Here we have the PVA, the length of the annuity, and the interest rate. We want to calculate the annuity

payment. Using the PVA equation and solving for the payment in each case, we find:

0

1

2

3

4

5

6

$24,500

C

PVA = C({1 – [1 / (1 + r)t]} / r))

$24,500 = $C{[1 – (1 / 1.11)6] / .11}

C = $24,500 / 4.23054

C = $5,791.23

0

1

2

3

4

5

6

7

8

$19,700

C

PVA = C({1 – [1 / (1 + r)t]} / r ))

CHAPTER 5 – 71

6. Here we need to find the present value of an annuity. Using the PVA equation, we find:

0

1

2

3

4

5

6

7

PVA

$2,100

PVA = C({1 – [1 / (1 + r)t]} / r)

PVA = $2,100{[1 – (1 / 1.05)7] / .05}

PVA = $12,151.38

0

1

2

3

4

5

6

7

8

9

PVA

$1,095

PVA = C({1 – [1 / (1 + r)t]} / r)

72 – SOLUTIONS

7. Here we have the FVA, the length of the annuity, and the interest rate. We want to calculate the annuity

payment. Using the FVA equation:

0

1

8

$30,000

C

FVA = C{[(1 + r)t – 1] / r}

$30,000 = $C[(1.058 – 1) / .05]

C = $30,000 / 9.54911

C = $3,141.65

0

1

…

40

$1,200,000

C

FVA = C{[(1 + r)t – 1] / r}

CHAPTER 5 – 73

8. Here, we need to find the future value of an annuity. Using the FVA equation, we find:

0

1

10

FVA

$1,900

FVA = C{[(1 + r)t – 1] / r}

FVA = $1,900[(1.0810 – 1) / .08]

FVA = $27,524.47

0

1

…

40

FVA

$6,000

9. Here we need to find the FVA. The equation to find the FVA is:

FVA = C{[(1 + r)t – 1] / r}

0

1

…

20

FVA

$5,000

74 – SOLUTIONS

10. The time line is:

0

1

∞

11. The time line is:

0

1

…

∞

–$645,000

$30,000

12. For discrete compounding, to find the EAR, we use the equation:

EAR = [1 + (APR / m)]m – 1

CHAPTER 5 – 75

13. Here we are given the EAR and need to find the APR. Using the equation for discrete compounding:

EAR = [1 + (APR / m)]m – 1

We can now solve for the APR. Doing so, we get:

14. For discrete compounding, to find the EAR, we use the equation:

EAR = [1 + (APR / m)]m – 1

So, for each bank, the EAR is:

15. The reported rate is the APR, so we need to convert the EAR to an APR as follows:

EAR = [1 + (APR / m)]m – 1

16. The time line is:

0

1

…

32

$1,345

FV

76 – SOLUTIONS

17. For this problem, we need to find the FV of a lump sum using the equation:

0

1

…

5(365)

$3,650

FV

FV in 5 years = $3,650[1 + (.047 / 365)]5(365) = $4,616.85

0

1

…

9(365)

PV

$65,000

For this problem, we simply need to find the PV of a lump sum using the equation:

PV = FV / (1 + r)t

APR = 164.40%

EAR = 3.6679, or 366.79%

20. The time line is:

0

1

…

60

$73,400

C

We first need to find the annuity payment. We have the PVA, the length of the annuity, and the interest

rate. Using the PVA equation:

78 – SOLUTIONS

21. The time line is:

0

1

…

t

–$14,480

$400

22. The time line is:

0

1

…

t

–$4

$5

Here we are trying to find the interest rate when we know the PV and FV. Using the FV equation:

FV = PV(1 + r)

23. The time line is:

0

1

…

∞

–$425,000

$3,300

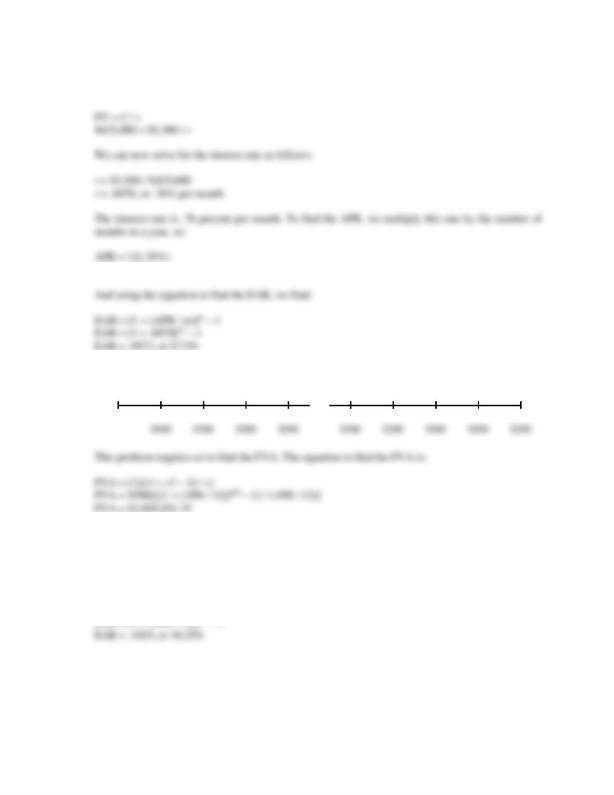
CHAPTER 5 – 79

Here we need to find the interest rate that equates the perpetuity cash flows with the PV of the cash

flows. Using the PV of a perpetuity equation:

APR = 9.32%

24. The time line is:

0

1

…

420

FVA

25. In the previous problem, the compounding period is monthly. This assumption still holds. Since the

cash flows are annual, we need to use the EAR to calculate the future value of annual cash flows. It is

important to remember that you have to make sure the compounding periods of the interest rate

matches with the cash flows. In this case, we have annual cash flows, so we need the EAR since it is

the true annual interest rate you will earn. So, finding the EAR:

EAR = [1 + (APR / m)]m – 1

80 – SOLUTIONS

26. The time line is:

0

1

…

16

PVA

$2,500

27. The time line is:

0

1

2

3

4

PV

$1,200

$1,100

$800

$600

28. The time line is:

0

1

2

3

4

PV

$1,400

$1,900

$3,400

$4,300

CHAPTER 5 – 81

29. The total interest paid by First Simple Bank is the interest rate per period times the number of periods.

In other words, the interest by First Simple Bank paid over 10 years will be:

30. The time line is:

0

1

…

60

$58,600

C

We need to use the PVA due equation, which is:

31. The time line is:

0

1

…

12

–$10,000

FV

Here we need to find the FV of a lump sum, with a changing interest rate. We must do this problem in

two parts. After the first six months, the balance will be:

82 – SOLUTIONS

32. We will calculate the time we must wait if we deposit in the bank that pays simple interest. The interest

amount we will receive each year in this bank will be:

Interest = $85,000(.048)

Interest = $4,080 per year

The deposit will have to increase by the difference between the amount we need by the amount we

33. The time line is:

0

1

…

12

–$1

FV

Here we need to find the future value of a lump sum. We need to make sure to use the correct number

of periods. So, the future value after one year will be:

CHAPTER 5 – 83

34. The time line is:

0

1

…

31

–£440

£60

35. Here we need to compare two cash flows. The only way to compare cash flows is to find the value of

the cash flows at a common time, so we will find the present value of each cash flow stream. Since

the cash flows are monthly, we need to use the monthly interest rate, which is:

Monthly rate = .07 / 12

Monthly rate = .0058 or .58%

The value today of the $6,700 monthly salary is:

0

1

…

24

PVA

$6,700

PVA = C({1 – [1/(1 + r)t]} / r )

PVA = $6,700{[1 – (1 / 1.0058)24] / .0058}

PVA = $149,645.17

To find the value of the second option, we find the present value of the monthly payments and add the

bonus. We can add the bonus since it is paid today. So:

84 – SOLUTIONS

0

1

…

24

$25,000

$5,400

PVA = C({1 – [1/(1 + r)t]} / r ))

PVA = $5,400{[1 – (1/1.0058)24] / .0058}

PVA = $120,609.54

So, the total value of the second option is:

Value of second option = $120,609.54 + 25,000

Value of second option = $145,609.54

The difference in the value of the two options today is:

Difference in value today = $149,645.17 – 145,609.54

Difference in value today = $4,035.63

What if we found the future value of the two cash flows? For the monthly salary, the future value will

be:

FVA = C{[(1 + r)t – 1] / r}

FVA = $6,700{[(1 + .0058)24 – 1] / .0058}

FVA = $172,062.91

To find the future value of the second option we also need to find the future value of the bonus as well.

So, the future value of this option is:

FV = C{[(1 + r)t – 1] / r} + PV(1 + r)t

FV = $5,400{[(1 + .0058)24 – 1] / .0058} + $25,000(1 + .0058)24

FV = $167,422.72

So, the first option is still the better choice. The difference between the future values of the two options

is:

Difference in future value = $172,062.91 – 167,422.72

Difference in future value = $4,640.19

No matter when you compare two cash flows, the cash flow with the greatest value in one period will

always have the greatest value in any other period. Here’s a question for you: What is the future value

of $4,035.63 (the difference in the cash flows at time zero) in 24 months at an interest rate of .58

36. The time line is:

0

1

…

20

PVA

$17,500

37. The investment we should choose is the investment with the higher rate of return. We will use the

future value equation to find the interest rate for each option. Doing so, we find the return for

Investment G is:

0

6

–$39,000

$75,000

FV = PV(1 + r)t

38. The time line is:

0

1

2

3

4

5

6

7

8

9

10

PVA

$7,300

The present value of an annuity falls as r increases, and the present value of an annuity rises as r

decreases. The future value of an annuity rises as r increases, and the future value of an annuity falls

as r decreases.

Here we need to calculate the present value of an annuity for different interest rates. Using the present

value of an annuity equation and an interest rate of 10 percent, we get:

86 – SOLUTIONS

39. The time line is:

0

1

2

3

4

5

6

7

…

t

–$50,000

$250

40. The time line is:

0

1

…

60

–$80,000

$1,650

Here we are given the PVA, number of periods, and the amount of the annuity. We need to solve for

the interest rate. Using the PVA equation:

PVA = ({1 – [1/(1 + r)t]} / r ))

$80,000 = $1,650[{1 – [1 / (1 + r)]60}/ r]

CHAPTER 5 – 87

41. The time line is:

0

1

2

3

4

5

PV

$3,400,000

$12,400,000

$13,400,000

42. The time line is:

0

1

2

3

4

5

6

$5,100,000

$985,000

$28,585,000

$15,085,000

$22,085,000

$24,085,000

$18,360,000

43. Here we are given the PVA, number of periods, and the amount of the annuity. We need to solve for

the interest rate. First, we need to find the amount borrowed since it is only 80 percent of the building

value. So, the amount borrowed is:

Amount borrowed = .80($2,950,000)

Amount borrowed = $2,360,000

0

1

…

360

–$2,360,000

$14,300

Now we can use the PVA equation:

PVA = C({1 – [1 / (1 + r)t]} / r))

$2,360,000 = $14,300[{1 – [1 / (1 + r)]360}/ r]

44. Here, we have two cash flow streams that will be combined in the future. In essence, we have three

time lines. We will start with the time lines for the savings period, which are:

Bond account:

0

1

2

3

4

5

6

7

8

9

10

$75,000

$6,000

Stock account:

0

10

$300,000

To find the withdrawal amount, we need to know the present value, as well as the interest rate and

periods, which are given. The present value of the retirement account is the future value of the stock

and bond account. We need to find the future value of each account and add the future values together.

For the bond account the future value is the value of the current savings plus the value of the annual

deposits. So, the future value of the bond account will be:

FV = C{[(1 + r)t – 1] / r} + PV(1 + r)t

CHAPTER 5 – 89

45. The time line is:

0

1

…

60

–$24,500

$465

Here we are given the PVA for an annuity due, number of periods, and the amount of the annuity. We

need to solve for the interest rate. Using the PVA equation:

46. a. If the payments are in the form of an ordinary annuity, the present value will be:

0

1

2

3

4

5

PVA

$13,500

PVA = C({1 – [1/(1 + r)t]} / r))

PVA = $13,500[{1 – [1 / (1 + .075)]5}/ .075]

PVA = $54,619.45

90 – SOLUTIONS

If the payments are an annuity due, the present value will be:

0

1

2

3

4

5

PVADue

$13,500

PVAdue = (1 + r) PVA

PVAdue = (1 + .075)$54,619.45

PVAdue = $58,715.90

b. We can find the future value of the ordinary annuity as:

47. Here, we need to find the difference between the present value of an annuity and the present value of

a perpetuity. The annuity time line is:

0

1

…

30

PVA

$12,700

PVA = C({1 – [1 / (1 + r)t]} / r))

PVA = $12,700{[1 – (1 / 1.054)30] / .054}

PVA = $186,634.92

CHAPTER 5 – 91

And the present value of the perpetuity is:

0

1

…

∞

PV

$12,700

PV = C / r

PV = $12,700 / .054

PV = $235,185.19

48. The time line is:

0

1

…

18

19

…

30

$7,750

Here we need to find the present value of an annuity at several different times. The annuity has

semiannual payments, so we need the semiannual interest rate. The semiannual interest rate is:

Semiannual rate = .09 / 2

Semiannual rate = .045

Now, we can use the present value of an annuity equation. Doing so, we get:

PVA = C({1 – [1/(1 + r)t]} / r))

PVA = $7,750{[1 – (1 / 1.045)12] / .045}

PVA = $70,669.00

92 – SOLUTIONS

49. The time line is:

0

1

6

…

20

PV

$2,150

Since the first payment is received six years from today and the last payment is received 20 years from

now, there are 15 payments. We can use the present value of an annuity formula, which will give us

50. The time line is:

0

1

…

120

PVA

$1,450

CHAPTER 5 – 93

Here, we have an annuity with two different interest rates. To answer this question, we simply need to

find the present value in multiple steps. The present value of the last six years’ payments at a 7 percent

interest rate is:

PVA = C({1 – [1 / (1 + r)t]} / r))

PVA = $1,450[{1 – 1 / [1 + (.07 / 12)]72} / (.07 / 12)]

PVA = $85,048.94

51. The time line is:

0

1

…

120

PVA

$1,145

To answer this question we need to find the future value of the annuity, and then find the present value

that makes the lump sum investment equivalent. We also need to make sure to use the number of

months as the number of periods. So, the future value of the annuity is:

94 – SOLUTIONS

52. The time line is:

0

1

…

19

20

…

∞

$5,000

Here we need to find the present value of a perpetuity at a date before the perpetuity begins. We will

begin by find the present value of the perpetuity. Doing so, we find:

53. The time line is:

0

1

…

12

–$25,000

$2,441.67

Here we are given the PVA, number of periods, and the amount of the annuity. We need to solve for

the interest rate. We must be careful to use the cash flows of the loan. Using the present value of an

annuity equation, we find:

PVA = C({1 – [1/(1 + r)t]} / r)

$25,000 = $2,441.67[{1 – [1 / (1 + r)]12}/ r]

CHAPTER 5 – 95

54. The time line is:

0

1

2

3

4

5

FV

$15,000

$24,000

$33,000

To solve this problem, we must find the FV of each cash flow and add them. To find the FV of a lump

sum, we use:

55. The payment for a loan repaid with equal payments is the annuity payment with the loan value as the

PV of the annuity. So, the loan payment will be:

PVA = C({1 – [1/(1 + r)t]} / r )

$54,000 = C{[1 – 1 / (1 + .08)3] / .08}

C = $20,953.81

3

19,401.68

20,953.81

1,552.13

19,401.68

0

96 – SOLUTIONS

56. This amortization table calls for equal principal payments of $18,000 per year. The interest payment is

3

18,000.00

19,440.00

1,440.00

18,000.00

0

In the third year, $1,440 of interest is paid.

57. The time line is:

0

1

–$15,570

$18,000

–24

–23

…

–12

–11

…

0

1

…

60

$3,583.33

$3,833.33

$4,083.33

$200,000

$25,000

Here we have cash flows that would have occurred in the past and cash flows that would occur in the

future. We need to bring both cash flows to today. Before we calculate the value of the cash flows

today, we must adjust the interest rate so we have the effective monthly interest rate. Finding the APR

CHAPTER 5 – 97

with monthly compounding and dividing by 12 will give us the effective monthly rate. The APR with

monthly compounding is:

APR = 12[(1.07)1/12 – 1]

APR = .0678, or 6.78%

To find the value today of the back pay from two years ago, we will find the FV of the annuity, and

then find the FV of the lump sum. Doing so gives us:

FVA = ($43,000 / 12)[{[1 + (.0678 / 12)]12 – 1} / (.0678 / 12)]

FVA = $44,362.73

59. Again, to find the interest rate of a loan, we need to look at the cash flows of the loan. Since this loan

is in the form of a lump sum, the amount you will repay is the FV of the principal amount, which will

be:

Loan repayment amount = $15,000(1.12) = $16,800

The amount you will receive today is the principal amount of the loan times one minus the points.

Amount received = $15,000(1 – .02) = $14,700

60. The time line is:

0

1

2

3

4

5

6

…

65

$800

$900

$1,000

FV

We need to find the FV of the premiums to compare with the cash payment promised at age 65. We

have to find the value of the premiums at Year 6 first since the interest rate changes at that time. So:

FV1 = $800(1.09)5 = $1,230.90

FV2 = $800(1.09)4 = $1,129.27

FV3 = $900(1.09)3 = $1,165.53

FV4 = $900(1.09)2 = $1,069.29

FV5 = $1,000(1.09)1 = $1,090.00

Value at Year 6 = $1,230.90 + 1,129.27 + 1,165.53 + 1,069.29 + 1,090 + 1,000

Value at Year 6 = $6,684.98

Finding the FV of this lump sum at the child’s 65th birthday:

FV = $6,684.98(1.055)59

FV = $157,396.57

The policy is not worth buying; the future value of the deposits is $157,396.57, but the policy contract

will pay off $150,000. The premiums are worth $7,396.57 more than the policy payoff.

Note, we could also compare the PV of the two cash flows. The PV of the premiums is:

PV = $800 / 1.09 + $800 / 1.092 + $900 / 1.093 + $900 / 1.094 + $1,000 / 1.095 + $1,000 / 1.096

PV = $3,986.04

CHAPTER 5 – 99

And the value today of the $150,000 at age 65 is:

PV = ($150,000 / 1.05559) / 1.096

PV = $3,798.72

The premiums still have the higher cash flow. At Time 0, the difference is $187.32. Whenever you are

comparing two or more cash flow streams, the cash flow with the highest value at one time will have

the highest value at any other time.

Here is a question for you: Suppose you invest $187.32, the difference in the cash flows at Time 0, for

six years at a 9 percent interest rate, and then for 59 years at a 5.5 percent interest rate. How much will

1.

CFo

$0

CFo

$0

CFo

$0

C01

$680

C01

$680

C01

$680

F01

1

F01

1

F01

1

2.

Enter

9

6%

$3,400

N

I/Y

PV

PMT

FV

Solve for

$23,125.75

Enter

5

6%

$5,200

100 – SOLUTIONS

3.

CFo

$0

CFo

$0

CFo

$0

C01

$985

C01

$985

C01

$985

F01

1

F01

1

F01

1

4.

Enter

15

8%

$5,450

N

I/Y

PV

PMT

FV

Solve for

$46,649.16

5.

Enter

6

11%

$24,500

N

I/Y

PV

PMT

FV

Solve for

$5,791.23

Enter

8

7%

$19,700

CHAPTER 5 – 101

6.

Enter

7

5%

$2,100

N

I/Y

PV

PMT

FV

Solve for

$12,151.38

Enter

9

10%

7.

Enter

8

5%

$30,000

N

I/Y

PV

PMT

FV

Solve for

$3,141.65

Enter

40

7%

8.

Enter

10

8%

$1,900

N

I/Y

PV

PMT

FV

Solve for

$27,524.47

Enter

40

9%

$6,000

102 – SOLUTIONS

Solve for

8.65%

104 – SOLUTIONS

22.

Enter

1

±$4

$5

CHAPTER 5 – 105

27.

CFo

$0

C01

$1,200

28.

CFo

$0

C01

$1,400

106 – SOLUTIONS

Enter

6

17.9% / 12

35.

Enter

24

7% /12

$6,700

36.

CHAPTER 5 – 107

38.

Enter

10

10%

$7,300

N

I/Y

PV

PMT

FV

Solve for

$44,855.34

40.

108 – SOLUTIONS

41.

42.

CFo

0

CFo

$5,100,000

C01

$3,400,000

C01

$985,000

F01

1

F01

1

C02

$12,400,000

C02

$28,585,000

F02

1

F02

1

43.

44. Future value of bond account:

Enter

10

10.5%

$300,000

CHAPTER 5 – 109

45. 2nd BGN 2nd SET

Enter

30

5.4%

$12,700

N

I/Y

PV

PMT

FV

Solve for

$186,634.92

And the present value of the perpetuity is:

110 – SOLUTIONS

48. Value at t = 9

Enter

12

9% / 2

$7,750

N

I/Y

PV

PMT

FV

Solve for

$70,669.00

Value at t = 5

Enter

4  2

9% / 2

$70,669.00

50. Value at t = 4

Enter

6  12

7% / 12

$1,450

CHAPTER 5 – 111

51. FV of A

Enter

10  12

7% / 12

$1,145

54.

CFo

$0

C01

$0

F01

1

112 – SOLUTIONS

Enter

12

6.78% / 12

$43,000 / 12

60. Value at Year 6:

Enter

5

9%

$800

N

I/Y

PV

PMT

FV

Solve for

$1,230.90

Enter

4

9%

$800

N

I/Y

PV

PMT

FV

Solve for

$1,129.27

CHAPTER 5 – 113

Enter

59

5.5%

CHAPTER 6

INTEREST RATES AND BOND VALUTION

Answers to Concepts Review and Critical Thinking Questions

3. No. If the bid were higher than the ask, the implication would be that a dealer was willing to sell a

bond and immediately buy it back at a higher price. How many such transactions would you like to

do?

5. There are two benefits. First, the company can take advantage of interest rate declines by calling in an

6. Bond issuers look at outstanding bonds of similar maturity and risk. The yields on such bonds are used

CHAPTER 6 – 115

11. As a general constitutional principle, the federal government cannot tax the states without their consent

15. a. The bond price is the present value when discounting the future cash flows from a bond; YTM is

the interest rate used in discounting the future cash flows (coupon payments and principal) back

to their present values.

b. If the coupon rate is higher than the required return on a bond, the bond will sell at a premium,

since it provides periodic income in the form of coupon payments in excess of that required by

investors on other similar bonds. If the coupon rate is lower than the required return on a bond,

the bond will sell at a discount, since it provides insufficient coupon payments compared to that

required by investors on other similar bonds. For premium bonds, the coupon rate exceeds the

YTM; for discount bonds, the YTM exceeds the coupon rate, and for bonds selling at par, the

116 – SOLUTIONS

1. The yield to maturity is the required rate of return on a bond expressed as a nominal annual interest

3. The price of any bond is the PV of the interest payment, plus the PV of the par value. Notice this

problem assumes an annual coupon. The price of the bond will be:

P = $70({1 – [1/(1 + .084)]9} / .084) + $1,000[1 / (1 + .084)9]

P = $913.98

We would like to introduce shorthand notation here. Rather than write (or type, as the case may be)

the entire equation for the PV of a lump sum, or the PVA equation, it is common to abbreviate the

equations as:

CHAPTER 6 – 117

4. Here, we need to find the YTM of a bond. The equation for the bond price is:

P = $961.50 = $70(PVIFAR%,9) + $1,000(PVIFR%,9)

Notice the equation cannot be solved directly for R. Using a spreadsheet, a financial calculator, or trial

and error, we find:

R = YTM = 7.61%

5. Here we need to find the coupon rate of the bond. All we need to do is to set up the bond pricing

equation and solve for the coupon payment as follows:

P = $963 = C(PVIFA6.14%,12) + $1,000(PVIF6.14%,12)

6. To find the price of this bond, we need to realize that the maturity of the bond is 14 years. The bond

118 – SOLUTIONS

7. Here, we are finding the YTM of a semiannual coupon bond. The bond price equation is:

8. Here, we need to find the coupon rate of the bond. All we need to do is to set up the bond pricing

equation and solve for the coupon payment as follows:

P = $945 = C(PVIFA3.1%,21) + $1,000(PVIF3.1%,21)

Solving for the coupon payment, we get:

9. The approximate relationship between nominal interest rates (R), real interest rates (r), and inflation

(h), is:

R = r + h

Approximate r = .045 – .016

Approximate r =.0285, or 2.85%

CHAPTER 6 – 119

13. The coupon rate, located in the second column of the quote is 6.125%. The bid price is:

Bid price = 146.1719%



$1,000

Bid price = $1,461.719

The previous day’s ask price is found by:

120 – SOLUTIONS

14. This is a premium bond because it sells for more than 100 percent of face value. The current yield is

based on the asked price, so the current yield is:

Current yield = Annual coupon payment / Price

15. To find the price of a zero coupon bond, we need to find the value of the future cash flows. With a

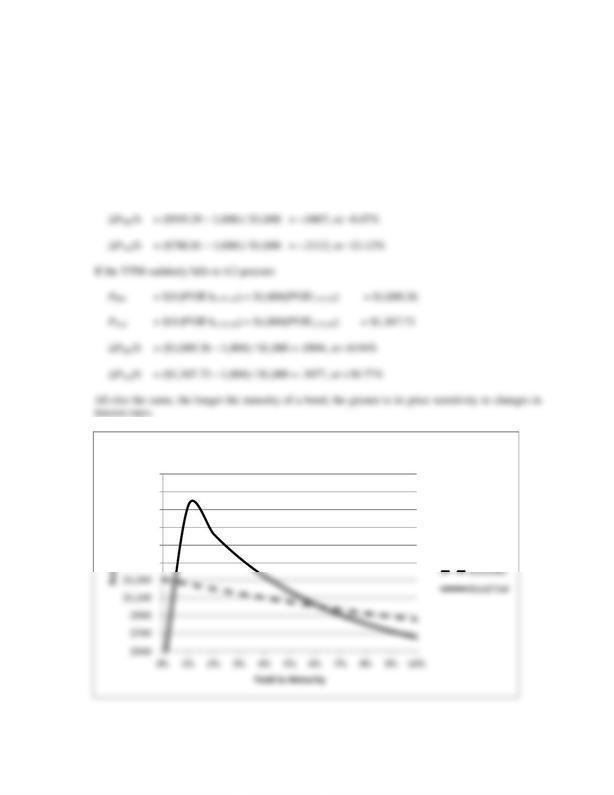
18. Here, we are finding the price of annual coupon bonds for various maturity lengths. The bond price

equation is:

P = C(PVIFAR%,t) + $1,000(PVIFR%,t)

X: P0 = $42.50(PVIFA3.5%,13) + $1,000(PVIF3.5%,13) = $1,126.68

P1 = $42.50(PVIFA3.5%,12) + $1,000(PVIF3.5%,12) = $1,120.44

P3 = $42.50(PVIFA3.5%,10) + $1,000(PVIF3.5%,10) = $1,106.59

P8 = $42.50(PVIFA3.5%,5) + $1,000(PVIF3.5%,5) = $1,062.37

P12 = $42.50(PVIFA3.5%,1) + $1,000(PVIF3.5%,1) = $1,014.25

P13 = $1,000

CHAPTER 6 – 121

$1,000

$1,100

$1,200

$1,300

Maturity and Bond Price

122 – SOLUTIONS

19. Any bond that sells at par has a YTM equal to the coupon rate. Both bonds sell at par, so the initial

YTM on both bonds is the coupon rate, 6.2 percent. If the YTM suddenly rises to 8.2 percent:

PBill = $31(PVIFA4.1%,10) + $1,000(PVIF4.1%,10) = $919.29

PTed = $31(PVIFA4.1%,50) + $1,000(PVIF4.1%,50) = $788.81

The percentage change in price is calculated as:

Percentage change in price = (New price – Original price) / Original price

$1,500

$1,700

$1,900

$2,100

$2,300

$2,500

YTM and Bond Price

CHAPTER 6 – 123

20. Initially, at a YTM of 8 percent, the prices of the two bonds are:

PJ = $20(PVIFA4%,26) + $1,000(PVIF4%,26) = $680.34

PS = $70(PVIFA4%,26) + $1,000(PVIF4%,26) = $1,479.48

If the YTM rises from 8 percent to 10 percent:

PJ = $20(PVIFA5%,26) + $1,000(PVIF5%,26) = $568.74

PS = $70(PVIFA5%,26) + $1,000(PVIF5%,26) = $1,287.50

The percentage change in price is calculated as:

21. The current yield is:

YTM = 6.56%

124 – SOLUTIONS

23. Accrued interest is the coupon payment for the period times the fraction of the period that has passed

since the last coupon payment. Since we have a semiannual coupon bond, the coupon payment per six

months is one-half of the annual coupon payment. There are five months until the next coupon

24. Accrued interest is the coupon payment for the period times the fraction of the period that has passed

since the last coupon payment. Since we have a semiannual coupon bond, the coupon payment per six

months is one-half of the annual coupon payment. There are two months until the next coupon

payment, so four months have passed since the last coupon payment. The accrued interest for the bond

is:

CHAPTER 6 – 125

YTM = 9.56%

26. a. The coupon bonds have coupon rate of 6.8 percent which matches the 6.8 percent required return,

so they will sell at par. The number of bonds that must be sold is the amount needed divided by

the bond price, so:

Number of coupon bonds to sell = $35,000,000 / $1,000

Number of coupon bonds to sell = 35,000

The number of zero coupon bonds to sell would be:

Price of zero coupon bonds = $1,000 / 1.03440

Price of zero coupon bonds = $262.53

Number of zero coupon bonds to sell = $35,000,000 / $262.53

Number of zero coupon bonds to sell = 133,318.247

b. The repayment of the coupon bond will be the par value plus the last coupon payment times the

number of bonds issued. So:

Coupon bonds repayment = 35,000($1,000) + 35,000($1,000)(.068 / 2)

Coupon bonds repayment = $36,190,000

The repayment of the zero coupon bond will be the par value times the number of bonds issued,

so:

Zeroes repayment = 133,318.247($1,000)

Zeroes repayment = $133,318,247

126 – SOLUTIONS

c. The total coupon payment for the coupon bonds will be the number bonds times the coupon

payment. For the cash flow of the coupon bonds, we need to account for the tax deductibility of

28. The bond asked price is 104.3850, so the dollar price is:

CHAPTER 6 – 127

29. The coupon rate of the bond is 6.125 percent and the bond matures in 20 years. The bond coupon

payments are semiannual, so the asked price is:

30. Here, we need to find the coupon rate of the bond. The price of the bond is:

Dollar price = 103.8235% × $1,000

Dollar price = $1,038.235

So the bond price equation is:

P = $1,038.235 = C(PVIFA1.09%,8) + $1,000(PVIF1.09%,8)

31. Here we need to find the yield to maturity. The dollar price of the bond is:

128 – SOLUTIONS

32. The bond price equation is:

33. Here, we need to find the coupon rate of the bond. The dollar price of the bond is:

Dollar price = 94.735% × $2,000

Dollar price = $1,894.70

Now, we need to do is to set up the bond pricing equation and solve for the coupon payment as follows:

P = $1,894.70 = C(PVIFA3.425%,24) + $2,000(PVIF3.425%,24)

34. To find the capital gains yield and the current yield, we need to find the price of the bond. The current

price of Bond P and the price of Bond P in one year is:

P: P0 = $85(PVIFA7%,5) + $1,000(PVIF7%,5) = $1,061.50

P1 = $85(PVIFA7%,4) + $1,000(PVIF7%,4) = $1,050.81

CHAPTER 6 – 129

Current yield = $85 / $1,061.50 = .0801, or 8.01%

The capital gains yield is:

Capital gains yield = (New price – Original price) / Original price

35. a. The rate of return you expect to earn if you purchase a bond and hold it until maturity is the YTM.

The bond price equation for this bond is:

P0 = $875 = $70(PVIFAR%,10) + $1,000(PVIF R%,10)

Using a spreadsheet, financial calculator, or trial and error we find:

R = YTM = 8.94%

b. To find our HPY, we need to find the price of the bond in two years. The price of the bond in two

years, at the new interest rate, will be:

P2 = $70(PVIFA7.94%,8) + $1,000(PVIF7.94%,8) = $945.70

130 – SOLUTIONS

Solve for

$27.40

CHAPTER 6 – 131

132 – SOLUTIONS

Solve for

$788.81

Enter

25 × 2

4.2% / 2

$62 / 2

$1,000

Solve for

$680.34

CHAPTER 6 – 133

PS

Enter

13 × 2

4%

Solve for

$568.74

PS

Enter

13 × 2

5%

$140 / 2

$1,000

Solve for

$821.23

PS

Enter

13 × 2

3%

134 – SOLUTIONS

CHAPTER 6 – 135

Solve for

$15.92

Solve for

$61.99

136 – SOLUTIONS

34.

Bond P

P0

Enter

5

7%

$85

$1,000

N

I/Y

PV

PMT

FV

Solve for

$1,061.50

Solve for

$938.50

Solve for

$949.19

Solve for

8.94%

CHAPTER 6 – 137

CHAPTER 7

EQUITY MARKETS AND STOCK

VALUATION

Answers to Concepts Review and Critical Thinking Questions

4. The general method for valuing a share of stock is to find the present value of all expected future

dividends. The dividend growth model presented in the text is only valid (i) if dividends are expected

CHAPTER 7 – 139

8. For a particular year, this can (and often does) happen. Going back to the cash flow identity, the

13. In a declassified board, every board seat is up for election every year. This structure allows investors

14. The major difficulty in using price ratio analysis is determining the correct benchmark PE ratio. In a

previous chapter, we showed how the sustainable growth rate is determined, and in a future chapter

we will discuss the required return. Although not exact measures, the growth rate and required return

have a solid economic basis. With the PE ratio, like any other ratio, it is difficult to determine what

140 – SOLUTIONS

1. The constant dividend growth model is:

Pt = Dt × (1 + g) / (R – g)

So, the price of the stock today is:

P0 = D0 (1 + g) / (R – g)

P0 = $2.35(1.041) / (.104 – .041)

P0 = $38.83

The dividend at Year 4 is the dividend today times the FVIF for the growth rate in dividends and four

years, so:

P3 = D3 (1 + g) / (R – g)

P3 = D0 (1 + g)4 / (R – g)

P3 = $2.35(1.041)4 / (.104 – .041)

P3 = $43.81

We can do the same thing to find the dividend in Year 16, which gives us the price in Year 15, so:

CHAPTER 7 – 141

3. The dividend yield is the dividend next year divided by the current price, so the dividend yield is:

4. Using the constant growth model, we find the price of the stock today is:

6. We know the stock has a required return of 11.5 percent, and the dividend and capital gains yields are

equal, so:

Dividend yield = 1/2(.115)

Dividend yield = .0575 = Capital gains yield

142 – SOLUTIONS

8. The price of a share of preferred stock is the dividend divided by the required return. This is the same

equation as the constant growth model, with a dividend growth rate of zero percent. Remember, most

9. If the company uses straight voting, you will need to own one-half of the shares, plus one share, in

order to guarantee enough votes to win the election. So, the number of shares needed to guarantee

election under straight voting will be:

Shares needed = (450,000 shares / 2) + 1

Shares needed = 225,001

And the total cost to you will be the shares needed times the price per share, or:

Total cost = 225,001  $34

Total cost = $7,650,034

CHAPTER 7 – 143

11. Here, we have a stock that pays no dividends for 20 years. Once the stock begins paying dividends, it

will have the same dividends forever, a preferred stock. We value the stock at that point, using the

preferred stock equation. It is important to remember that the price we find will be the price one year

before the first dividend, so:

12. Here, we need to value a stock with two different required returns. Using the constant growth model

and a required return of 12 percent, the stock price today is:

P0 = D1 / (R – g)

P0 = $3.14 / (.12 – .045)

P0 = $41.87

144 – SOLUTIONS

13. Using the equation to calculate the price of a share of stock with the PE ratio:

P = Benchmark PE ratio × EPS

So, with a PE ratio of 18, we find:

14. First, we need to find the sales per share, which is:

9. The dividend growth model is similar to the present value of an annuity and the present value of a

perpetuity: The equation gives you the present value one period before the first payment. So, the price

of the stock in Year 9 will be:

CHAPTER 7 – 145

17. With nonconstant dividends, we find the price of the stock when the dividends level off at a constant

growth rate, and then find the present value of the future stock price, plus the present value of all

dividends during the nonconstant growth period. The stock begins constant growth after the fourth

dividend is paid, so we can find the price of the stock at Year 4, when the constant dividend growth

begins, as:

18. With nonconstant dividends, we find the price of the stock when the dividends level off at a constant

growth rate, and then find the present value of the future stock price, plus the present value of all

dividends during the nonconstant growth period. The stock begins constant growth after the third

dividend is paid, so we can find the price of the stock in Year 3, when the constant dividend growth

begins as:

146 – SOLUTIONS

20. We are given the stock price, the dividend growth rate, and the required return, and are asked to find

the dividend. Using the constant dividend growth model, we get:

21. The highest dividend yield will occur when the stock price is the lowest. So, using the 52-week low

stock price, the highest dividend yield was:

22. With nonconstant dividends, we find the price of the stock when the dividends level off at a constant

growth rate, and then find the present value of the future stock price, plus the present value of all

dividends during the nonconstant growth period. Since the first dividend with constant growth is in

Year 6, we can find the price of the stock in Year 5, one year before the constant dividend growth

begins as:

P5 = D6 / (R – g)

P5 = D0 (1 + g1)5 (1 + g2) / (R – g)

CHAPTER 7 – 147

23. We need to find the required return of the stock. Using the constant growth model, we can solve the

equation for R. Doing so, we find:

24. We need to find the required return of the stock. Using the constant growth model, we can solve the

equation for R. Doing so, we find:

25. The annual dividend paid to stockholders is $.31, and the dividend yield is .90 percent. Using the

equation for the dividend yield:

Dividend yield = Dividend / Stock price

We can plug the numbers in and solve for the stock price:

.009 = $.31 / P0

148 – SOLUTIONS

26. a. Using the equation to calculate the price of a share of stock with the PE ratio:

P = Benchmark PE ratio × EPS

So, with a PE ratio of 19, we find:

P = 19($4.65)

P = $88.35

b. First, we need to find the earnings per share next year, which will be:

EPS1 = EPS0(1 + g)

EPS1 = $4.65(1 + .07)

EPS1 = $4.98

27. We need to find the PE ratio each year, which is:

PE1 = $53.18 / $3.15 = 16.88

PE2 = $62.14 / $3.35 = 18.55

PE3 = $73.21 / $3.60 = 20.34

PE4 = $70.21 / $3.85 = 18.24

So, the average PE is:

CHAPTER 7 – 149

28. First, we need to find the earnings per share next year, which will be:

EPS1 = EPS0(1 + g)

EPS1 = $2.95(1 + .09)

EPS1 = $3.22

To find the high target stock price, we need to find the average high PE ratio each year, which is:

P1 = 23.90($3.22)

P1 = $76.84

To find the low target stock price, we need to find the average low PE ratio each year, which is:

PE1 = $37.25 / $2.35 = 15.85

150 – SOLUTIONS

29. To find the target price in five years, we first need to find the EPS in five years, which will be:

EPS5 = EPS0(1 + g)5

30. We need to begin by finding the dividend for each year over the next five years, so:

D1 = $1.15(1 + .10) = $1.27

D2 = $1.15(1 + .10)2 = $1.39

D3 = $1.15(1 + .10)3 = $1.53

D4 = $1.15(1 + .10)4 = $1.69

D5 = $1.15(1 + .10)5 = $1.86

To find the EPS in Year 5, we can use the dividends and payout ratio, which gives us:

EPS5 = D5 / Payout ratio

CHAPTER 7 – 151

31. We are asked to find the dividend yield and capital gains yield for each of the stocks. All of the stocks

have a required return of 17 percent, which is the sum of the dividend yield and the capital gains yield.

To find the components of the total return, we need to find the stock price for each stock. Using this

stock price and the dividend, we can calculate the dividend yield. The capital gains yield for each stock

will be the total return (required return) minus the dividend yield.

W: P0 = D0(1 + g) / (R – g)

P0 = $2.40(1.08) / (.17 – .08)

P0 = $28.80

Dividend yield = D1/P0

Dividend yield = $2.40(1.08) / $28.80

Dividend yield = .09, or 9%

Capital gains yield = Total return – Dividend yield

Capital gains yield = .17 – .09

Capital gains yield = .08, or 8%

X: P0 = D0(1 + g) / (R – g)

P0 = $2.40 / (.17 – .00)

P0 = $14.12

Dividend yield = D1 / P0

Dividend yield = $2.40 / $14.12

Dividend yield = .17, or 17%

Capital gains yield = Total return – Dividend yield

Capital gains yield = .17 – .17

Capital gains yield = .00, or 0%

Y: P0 = D0(1 + g) / (R – g)

P0 = $2.40(1 – .05) / [.17 – (–.05)]

P0 = $10.36

152 – SOLUTIONS

32. a. Using the constant growth model, the price of the stock paying annual dividends will be:

P0 = D0(1 + g) / (R – g)

P0 = $2.20(1.06) / (.12 – .06)

P0 = $38.87

b. If the company pays quarterly dividends instead of annual dividends, the quarterly dividend will

be one-fourth of annual dividend, or:

Quarterly dividend = $2.20(1.06) / 4

Quarterly dividend = $.5830

To find the equivalent annual dividend, we must assume that the quarterly dividends are

reinvested at the required return. We can then use this interest rate to find the equivalent annual

dividend. In other words, when we receive the quarterly dividend, we reinvest it at the required

CHAPTER 7 – 153

CHAPTER 8

NET PRESENT VALUE AND OTHER

INVESTMENT CRITERIA

Answers to Concepts Review and Critical Thinking Questions

3. a. Payback period is simply the break-even point of a series of cash flows. To actually compute the

payback period, it is assumed that any cash flow occurring during a given period is realized

continuously throughout the period, and not at a single point in time. The payback is then the

point in time for the series of cash flows when the initial cash outlays are fully recovered. Given

some predetermined cutoff for the payback period, the decision rule is to accept projects that

payback before this cutoff, and reject projects that take longer to payback.

4. a. The average accounting return is interpreted as an average measure of the accounting

performance of a project over time, computed as some average profit measure due to the project

divided by some average balance sheet value for the project. This text computes AAR as average

net income with respect to average (total) book value. Given some predetermined cutoff for AAR,

the decision rule is to accept projects with an AAR in excess of the target measure, and reject all

other projects.

5. a. NPV is simply the sum of the present values of a project’s cash flows. NPV specifically measures,

after considering the time value of money, the net increase or decrease in firm wealth due to the

project. The decision rule is to accept projects that have a positive NPV, and reject projects with

a negative NPV.

6. a. The IRR is the discount rate that causes the NPV of a series of cash flows to be equal to zero.

IRR can thus be interpreted as a financial break-even rate of return; at the IRR discount rate, the

net value of the project is zero. The IRR decision rule is to accept projects with IRRs greater than

the discount rate, and to reject projects with IRRs less than the discount rate.

7. a. The profitability index is the present value of the future cash flows divided by the initial

investment. As such, it is a benefit/cost ratio, providing a measure of the relative profitability of

156 – SOLUTIONS

8. For a project with future cash flows that are an annuity:

Payback = I / C

And the IRR is:

0 = – I + C / IRR

9. There are a number of reasons. Two of the most important have to do with transportation costs and

exchange rates. Manufacturing in the U.S. places the finished product much closer to the point of sale,

11. Yes, they are. Such entities generally need to allocate available capital efficiently, just as for-profits

CHAPTER 8 – 157

13. The MIRR is calculated by finding the present value of all cash outflows, the future value of all cash

14. The statement is incorrect. It is true that if you calculate the future value of all intermediate cash flows

to the end of the project at the required return, then calculate the NPV of this future value and the

initial investment, you will get the same NPV. However, NPV says nothing about reinvestment of

intermediate cash flows. The NPV is the present value of the project cash flows. What is actually done

with those cash flows once they are generated is not relevant. Put differently, the value of a project

15. The statement is incorrect. It is true that if you calculate the future value of all intermediate cash flows

to the end of the project at the IRR, then calculate the IRR of this future value and the initial

investment, you will get the same IRR. However, as in the previous question, what is done with the

cash flows once they are generated does not affect the IRR. Consider the following example:

C0

C1

C2

IRR

Project A

–$100

$10

$110

10%

Suppose this $100 is a deposit into a bank account. The IRR of the cash flows is 10 percent. Does the

158 – SOLUTIONS

1. To calculate the payback period, we need to find the time it takes to recover the initial investment.

After two years, the project has created:

$2,800 + 3,200 = $6,000

in cash flows. The project still needs to create another:

2. To calculate the payback period, we need to find the time it takes to recover the initial investment. The

cash flows in this problem are an annuity, so the calculation is simpler. If the initial cost is $3,100, the

payback period is:

Payback = 3 + $295 / $935

Payback = 3.32 years

There is a shortcut to calculate payback period when the future cash flows are an annuity. Just divide

the initial cost by the annual cash flow. For the $3,100 cost, the payback period is:

Payback = $3,100 / $935

Payback = 3.22 years

For an initial cost of $4,300, the payback period is:

CHAPTER 8 – 159

3. Project A has cash flows of:

Cash flows = $23,000 + 28,000

Cash flows = $51,000

during the first two years. The cash flows are still short by $9,000 of recapturing the initial investment,

so the payback for Project A is:

4. Our definition of AAR is the average net income divided by the average book value. The average net

income for this project is:

Average net income = ($1,368,000 + 1,935,000 + 1,738,000 + 1,310,000) / 4

Average net income = $1,587,750

And the average book value is:

160 – SOLUTIONS

6. The NPV of a project is the PV of the outflows minus by the PV of the inflows. The equation for the

NPV of this project at a 9 percent required return is:

7. The NPV of a project is the PV of the outflows plus the PV of the inflows. Since the cash inflows are

an annuity, the equation for the NPV of this project at an 8 percent required return is:

NPV = –$8,450 + $2,145(PVIFA8%, 8)

NPV = $3,876.54

At an 8 percent required return, the NPV is positive, so we would accept the project.

The equation for the NPV of the project at a 24 percent required return is:

CHAPTER 8 – 161

9. The NPV of a project is the PV of the outflows plus by the PV of the inflows. At a zero discount rate

(and only at a zero discount rate), the cash flows can be added together across time. So, the NPV of

the project at a zero percent required return is:

NPV = –$19,400 + 9,800 + 11,300 + 6,900

NPV = $8,600

The NPV at a 10 percent required return is:

NPV = –$19,400 + $9,800 / 1.10 + $11,300 / 1.102 + $6,900 / 1.103

NPV = $4,032.01

162 – SOLUTIONS

10. a. The IRR is the interest rate that makes the NPV of the project equal to zero. The equation for the

IRR of Project A is:

IRR = 20.70%

The equation for the IRR of Project B is:

IRR = 18.73%

Examining the IRRs of the projects, we see that the IRRA is greater than the IRRB, so the IRR

decision rule implies accepting Project A. This may not be a correct decision, however, because

the IRR criterion has a ranking problem for mutually exclusive projects. To see if the IRR

decision rule is correct or not, we need to evaluate the project NPVs.

b. The NPV of Project A is:

NPVA = –$78,500 + $43,000 / 1.11+ $29,000 / 1.112 + $23,000 / 1.113 + $21,000 / 1.114

NPVA = $14,426.54

R = 12.21%

IRR = 15.98%

IRR = 14.95%

R = 7.62%

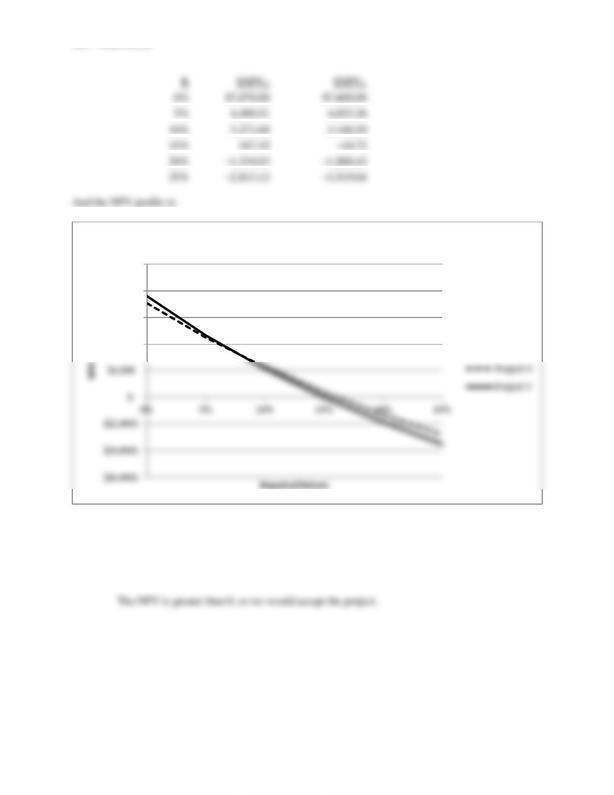
164 – SOLUTIONS

12. a. The equation for the NPV of the project is:

NPV = –$39,000,000 + $57,000,000 / 1.10 – $9,000,000 / 1.102

NPV = $5,380,165.29

$4,000

$6,000

$8,000

$10,000

NPV Profile

CHAPTER 8 – 165

IRR = 28.15%, –81.99%

PI = 1.072

The equation for the profitability index at a required return of 22 percent is:

PIII = 1.187

The profitability index decision rule implies that we accept Project II, since PIII is greater than

the PII.

166 – SOLUTIONS

b. The NPV of each project is:

15. a. The payback period for each project is:

A: 3 + ($110,000 / $325,000) = 3.34 years

B: 1 + ($18,300 / $19,900) = 1.92 years

The payback criterion implies accepting Project B, because it pays back sooner than Project A.

b. The NPV for each project is:

IRR = 21.02%

IRR = 30.57%

PI = 1.338

Profitability index criterion implies accept Project B because its PI is greater than Project A’s.

16. a. The IRR for each project is:

IRR = 27.82%

IRR = 25.57%

IRR decision rule implies we accept Project M because IRR for M is greater than IRR for N.

b. The NPV for each project is:

M: NPV = –$150,000 + $68,600 / 1.15 + $76,800 / 1.152 + $71,300 / 1.153 + $40,500 / 1.154

NPV = $37,760.92

168 – SOLUTIONS

PI = 1.166

Profitability index criterion implies accept Project Y because its PI is greater than Project Z’s.

b. The NPV for each project is:

18. To find the crossover rate, we subtract the cash flows from one project from the cash flows of the other

R = 14.06%

20. At a zero discount rate (and only at a zero discount rate), the cash flows can be added together across

time. So, the NPV of the project at a zero percent required return is:

NPV = –$745,382 + 265,381 + 304,172 + 225,153 + 208,614

NPV = $257,938

CHAPTER 8 – 169

21. a. The payback period for each project is:

F: 2 + ($21,600 / $81,600) = 2.26 years

G: 3 + ($5,200 / $166,800) = 3.03 years

The payback criterion implies accepting Project F because it pays back sooner than Project G.

Project G does not meet the minimum payback of three years.

b. The NPV for each project is:

F: NPV = –$180,000 + $93,600 / 1.10 + $64,800 / 1.102 + $81,600 / 1.103 + $72,000 / 1.104

170 – SOLUTIONS

22. The MIRR for the project with all three approaches is:

Discounting approach:

In the discounting approach, we find the value of all cash outflows to Time 0, while any cash inflows

remain at the time at which they occur. So, discounting the cash outflows to Time 0, we find:

Time 0 cash flow = –$27,500 – $4,050 / 1.105

Time 0 cash flow = –$30,014.73

So, the MIRR using the discounting approach is:

0 = –$30,014.73 + $10,430 / (1 + MIRR) + $13,850 / (1 + MIRR)2 + $11,270 / (1 + MIRR)3

+ $9,830 / (1 + MIRR)4

Using a spreadsheet, financial calculator, or trial and error to find the root of the equation, we find that:

MIRR = 19.21%

Reinvestment approach:

In the reinvestment approach, we find the future value of all cash except the initial cash flow at the

end of the project. So, reinvesting the cash flows to Time 5, we find:

Time 5 cash flow = $10,430(1.104) + $13,850(1.103) + $11,270(1.102) + $9,830(1.10) – $4,050

Time 5 cash flow = $54,104.61

CHAPTER 8 – 171

23. With different discounting and reinvestment rates, we need to make sure to use the appropriate interest

rate. The MIRR for the project with all three approaches is:

Discounting approach:

In the discounting approach, we find the value of all cash outflows to Time 0 at the discount rate, while

any cash inflows remain at the time at which they occur. So, discounting the cash outflows to Time 0,

we find:

Time 0 cash flow = –$27,500 – $4,050 / 1.115

Time 0 cash flow = –$29,903.48

So, the MIRR using the discounting approach is:

0 = –$29,903.48 + $10,430 / (1 + MIRR) + $13,850 / (1 + MIRR)2 + $11,270 / (1 + MIRR)3

+ $9,830 / (1 + MIRR)4

Using a spreadsheet, financial calculator, or trial and error to find the root of the equation, we find that:

MIRR = 19.41%

Reinvestment approach:

In the reinvestment approach, we find the future value of all cash except the initial cash flow at the

end of the project using the reinvestment rate. So, reinvesting the cash flows to Time 5, we find:

172 – SOLUTIONS

24. To find the crossover rate, we subtract the cash flows from one project from the cash flows of the other

R = 9.24%

The NPV of the projects at the crossover rate must be equal. The NPV of each project at the crossover

rate is:

IRR = 7.47%

At an interest rate of 10 percent, the NPV is:

NPV = $91,000 – $55,000 / 1.10 – $46,000 / 1.102

NPV = $2,983.47

At an interest rate of zero percent, we can add cash flows, so the NPV is:

CHAPTER 8 – 173

26. By definition, the profitability index is:

PI = Discounted Value of Future Cash Flows / Initial Cost

But note that the discounted value of future cash flows is just the NPV overstated by the neglected

27. a. To have a payback equal to the project’s life, given C is a constant cash flow for N years:

C = I/N

174 – SOLUTIONS

28. a. Here the cash inflows of the project go on forever, which is a perpetuity. Unlike ordinary

perpetuity cash flows, the cash flows here grow at a constant rate forever, which is a growing

perpetuity. If you remember back to the chapter on stock valuation, we presented a formula for

valuing a stock with constant growth in dividends. This formula is actually the formula for a

growing perpetuity, so we can use it here. The PV of the future cash flows from the project is:

PV of cash inflows = C1 / (R – g)

PV of cash inflows = $135,000 / (.12 – .047)

PV of cash inflows = $1,849,315.07

29. The IRR is the interest rate that makes the NPV of the project equal to zero. So, the IRR of the project

is:

0 = $35,000 – $27,000 / (1 + IRR) + $29,000 / (1 + IRR)2

Even though it appears there are two IRRs, a spreadsheet, financial calculator, or trial and error will

not give an answer. The reason is that there is no real IRR for this set of cash flows. If you examine

the IRR equation, what we are really doing is solving for the roots of the equation. Going back to high

school algebra, in this problem we are solving a quadratic equation. In case you don’t remember, the

quadratic formula is:

2−−

CHAPTER 8 – 175

30. First, we need to find the future value of the cash flows for the one year in which they are blocked by

the government. So, reinvesting each cash inflow for one year, we find:

Year 2 cash flow = $303,800(1.04) = $315,952

Year 3 cash flow = $219,700(1.04) = $228,488

Year 4 cash flow = $320,000(1.04) = $332,800

Year 5 cash flow = $288,700(1.04) = $300,248

IRR = 9.42%

5.

CFo

–$168,500

176 – SOLUTIONS

6.

CFo

–$168,500

CFo

–$168,500

C01

$86,000

C01

$86,000

8.

CFo

–$19,400

9.

CFo

–$19,400

CFo

–$19,400

C01

$9,800

C01

$9,800

F01

1

F01

1

C02

$11,300

C02

$11,300

F02

1

F02

1

C03

$6,900

C03

$6,900

F03

1

F03

1

CHAPTER 8 – 177

10.

CF (A)

Cfo

–$78,500

CFo

–$78,500

C01

$43,000

C01

$43,000

20.70%

NPV CPT

$14,426.54

CF (B)

CFo

–$78,500

CFo

–$78,500

18.73%

NPV CPT

$15,012.82

178 – SOLUTIONS

Crossover rate:

CFo

$0

11.

CF (X)

CFo

–$23,400

CFo

–$23,400

C01

$13,100

C01

$13,100

F01

1

F01

1

C02

$9,480

C02

$9,480

F02

1

F02

1

C03

$7,890

C03

$7,890

F03

1

F03

1

I = 0%

I = 25%

NPV CPT

$7,070

–$2,813.12

CF (Y)

CFo

–$23,400

CFo

–$23,400

CHAPTER 8 – 179

12.

13.

CFo

$0

CFo

$0

CFo

$0

C01

$15,800

C01

$15,800

C01

$15,800

F01

1

F01

1

F01

1

C02

$13,600

C02

$13,600

C02

$13,600

14. a. The profitability indexes are:

CF (I)

CF (II)

CFo

$0

CFo

$0

C01

$28,300

C01

$9,600

F01

1

F01

1

C02

$34,800

C02

$17,400

F02

1

F02

1

C03

$43,700

C03

$15,600

F03

1

F03

1

I = 11%

NPV CPT

$85,693.02

$34,177.46

180 – SOLUTIONS

15.

CF (A)

CFo

–$235,000

CFo

–$235,000

CFo

$0

C01

$29,000

C01

$29,000

C01

$29,000

NPV CPT

21.02%

NPV CPT

$60,579.46

$295,579.46

PI = $295,579.46 / $235,000 = 1.258

CF (B)

CFo

–$47,000

CFo

–$47,000

CFo

$0

CHAPTER 8 – 181

16.

Project M

CFo

–$150,000

CFo

–$150,000

C01

$68,600

C01

$68,600

27.82%

NPV CPT

$37,760.92

Project N

CFo

–$372,000

CFo

–$372,000

17.

Project Y

CFo

$0

CFo

–$43,400

C01

$19,800

C01

$19,800

F01

1

F01

1

C02

$17,500

C02

$17,500

F02

1

F02

1

C03

$20,700

C03

$20,700

F03

1

F03

1

C04

$14,600

C04

$14,600

F04

1

F04

1

I = 12%

NPV CPT

$55,641.88

$12,241.88

182 – SOLUTIONS

18.

CFo

$0

C01

$23,200

20.

CFo

–$745,382

CFo

–$745,382

C01

$265,381

C01

$265,381

CHAPTER 8 – 183

21. b.

Project F

Project G

CFo

–$180,000

CFo

–$280,000

C01

$93,600

C01

$64,800

F01

1

F01

1

C02

$64,800

C02

$86,400

24. Crossover rate:

CFo

$31,000

C01

–$3,000

F01

1

9.24%

Project R

Project S

CFo

–$45,000

CFo

–$76,000

C01

$17,000

C01

$20,000

184 – SOLUTIONS

25.

7.47%

CFo

$91,000

CFo

$91,000

CFo

$91,000

CHAPTER 9

MAKING CAPITAL INVESTMENT

DECISIONS

Answers to Concepts Review and Critical Thinking Questions

6. There are two particularly important considerations. The first is erosion. Will the essentialized book

simply displace copies of the existing book that would have otherwise been sold? This is of special

concern given the lower price. The second consideration is competition. Will other publishers step in

186 – SOLUTIONS

14. The option to abandon reflects our ability to reallocate assets if we find our initial estimates were too

optimistic. The option to expand reflects our ability to increase cash flows from a project if we find

our initial estimates were too pessimistic. Since the option to expand can increase cash flows and the

1. The $5.3 million acquisition cost of the land six years ago is a sunk cost. The $7.4 million current

2. Sales due solely to the new product line are:

29,000($23,500) = $681,500,000

CHAPTER 9 – 187

Increased sales of the motor home line occur because of the new product line introduction; thus:

3. We need to construct an income statement. The income statement is:

Sales $ 645,000

4. To find the OCF, we need to complete the income statement as follows:

Sales $ 558,400

Variable costs 346,800

Depreciation 94,500

EBIT $ 117,100

Taxes@35% 40,985

188 – SOLUTIONS

5. The MACRS depreciation schedule is shown in Table 9.7. The ending book value for any year is the

6. The asset has a useful life of 8 years and we want to find the book value of the asset after 5 years. With

straight-line depreciation, the depreciation each year will be:

Annual depreciation = $545,000 / 8

Annual depreciation = $68,125

So, after five years, the accumulated depreciation will be:

Accumulated depreciation = 5($68,125)

Accumulated depreciation = $340,625

CHAPTER 9 – 189

7. To find the book value at the end of four years, we need to find the accumulated depreciation for the

first four years. We could calculate a table as in Problem 5, but an easier way is to add the MACRS

Lost sales of old

–8,217,000

Variable costs

18,003,150

Fixed costs

3,200,000

Depreciation

2,150,000

190 – SOLUTIONS

11. The cash outflow at the beginning of the project will increase because of the spending on NWC. At

the end of the project, the company will recover the NWC, so it will be a cash inflow. The sale of the

equipment will result in a cash inflow, but we must also account for the taxes that will be paid on this

sale. So, the cash flows for each year of the project will be:

12. First, we will calculate the annual depreciation for the equipment necessary for the project. The

depreciation amount each year will be:

Year 1 depreciation = $1,950,000(.3333) = $649,935

Year 2 depreciation = $1,950,000(.4445) = $866,775

Year 3 depreciation = $1,950,000(.1481) = $288,795

So, the book value of the equipment at the end of three years, which will be the initial investment

minus the accumulated depreciation, is:

Book value in 3 years = $1,950,000 – ($649,935 + 866,775 + 288,795)

Book value in 3 years = $144,495

The asset is sold at a gain to book value, so this gain is taxable.

CHAPTER 9 – 191

13. First, we will calculate the annual depreciation of the new equipment. It will be:

Annual depreciation = $655,000 / 5

Annual depreciation = $131,000

Now, we calculate the aftertax salvage value. The aftertax salvage value is the market price minus (or

plus) the taxes on the sale of the equipment, so:

Aftertax salvage value = MV + (BV – MV)TC

Very often, the book value of the equipment is zero, as it is in this case. If the book value is zero, the

equation for the aftertax salvage value becomes:

14. First, we will calculate the annual depreciation of the new equipment. It will be:

Annual depreciation charge = $410,000 / 5

Annual depreciation charge = $82,000

The aftertax salvage value of the equipment is:

Aftertax salvage value = $30,000(1 – .35)

Aftertax salvage value = $19,500

192 – SOLUTIONS

15. To evaluate the project with a $145,000 cost savings, we need the OCF to compute the NPV. Using

the tax shield approach, the OCF is:

OCF = $145,000(1 – .35) + .35($82,000)

OCF = $122,950

16. The base-case, best-case, and worst-case values are shown below. Remember that in the best-case,

sales and price increase, while costs decrease. In the worst case, sales and price decrease, and costs

increase.

CHAPTER 9 – 193

17. An estimate for the impact of changes in price on the profitability of the project can be found from the

18. a. We will use the tax shield approach to calculate the OCF. The OCF is:

OCFbase = [(P – v)Q – FC](1 – TC) + Depreciation(TC)

OCFbase = [($37.95 – 23.20)(91,000) – $815,000](.65) + .35($1,720,000 / 6)

OCFbase = $443,045.83

Now we can calculate the NPV using our base-case projections. There is no salvage value or

NWC, so the NPV is:

NPVbase = –$1,720,000 + $443,045.83(PVIFA11%,6)

NPVbase = $154,322.17

To calculate the sensitivity of the NPV to changes in the quantity sold, we will calculate the NPV

at a different quantity. We will use sales of 92,000 units. The NPV at this sales level is:

OCFnew = [($37.95 – 23.20)(92,000) – $815,000](.65) + .35($1,720,000 / 6)

OCFnew = $452,633.33

And the NPV is:

NPVnew = –$1,720,000 + $452,633.33(PVIFA11%,6)

NPVnew = $194,882.45

So, the change in NPV for every unit change in sales is:

194 – SOLUTIONS

19. We will use the tax shield approach to calculate the OCF for the best- and worst-case scenarios. For

the best-case scenario, the price and quantity increase by 10 percent, so we will multiply the base case

numbers by 1.1, a 10 percent increase. The variable and fixed costs both decrease by 10 percent, so

we will multiply the base case numbers by .9, a 10 percent decrease. Doing so, we get:

OCFbest = {[$37.95(1.1) – $23.20(.9)](91,000)(1.1) – $815,000(.9)}(.65) + .35($1,720,000 / 6)

OCFbest = $981,139.56

The best-case NPV is:

CHAPTER 9 – 195

20. First, we need to calculate the cash flows. The marketing study is a sunk cost and should be ignored.

The net income each year will be:

Sales of new product

$815,000

Variable costs

163,000

Fixed costs

196,000

Depreciation

216,250

EBT

$239,750

Tax

95,900

Net income

$143,850

21. First, we will calculate the depreciation each year, which will be:

D1 = $375,000(.2000) = $75,000

D2 = $375,000(.3200) = $120,000

D3 = $375,000(.1920) = $72,000

D4 = $375,000(.1152) = $43,200

The book value of the equipment at the end of the project is:

BV4 = $375,000 – ($75,000 + 120,000 + 72,000 + 43,200)

BV4 = $64,800

196 – SOLUTIONS

The asset is sold at a loss to book value, so this creates a tax refund.

Aftertax salvage value = $45,000 + ($64,800 – 45,000)(.34)

Aftertax salvage value = $51,732

Using the depreciation tax shield approach, the OCF for each year will be:

OCF1 = $142,000(1 – .34) + .34($75,000) = $119,220

OCF2 = $142,000(1 – .34) + .34($120,000) = $134,520

OCF3 = $142,000(1 – .34) + .34($72,000) = $118,200

OCF4 = $142,000(1 – .34) + .34($43,200) = $108,408

Now, we have all the necessary information to calculate the project NPV. We need to be careful with

the NWC in this project. Notice the project requires $15,000 of NWC at the beginning, and $2,000

more in NWC each successive year. We will subtract the $15,000 from the initial cash flow, and

subtract $2,000 each year from the OCF to account for this spending. In Year 4, we will add back the

total spent on NWC, which is $21,000. The $2,000 spent on NWC capital during Year 4 is irrelevant.

22. Using the tax shield approach, the OCF at 90,000 units will be:

OCF = [(P – v)Q – FC](1 – TC) + Depreciation(TC)

OCF = [($38.70 – 29.65)(90,000) – 315,000](.66) + .34($645,000 / 5)

OCF = $373,530

We will calculate the OCF at 91,000 units. The choice of the second level of quantity sold is arbitrary

CHAPTER 9 – 197

23. a. The base-case, best-case, and worst-case values are shown below. Remember that in the best-

case, unit sales increase, while fixed and variable costs decrease. In the worst case, unit sales

decrease, while fixed and variable costs increase.

Scenario Unit sales Variable cost Fixed costs

Base 180 $11,100 $535,000

Best 198 $9,990 $481,500

Worst 162 $12,120 $588,500

Using the tax shield approach, the OCF and NPV for the base case estimate is:

OCFbase = [($16,300 – 11,100)(180) – $535,000](.65) + .35($780,000 / 4)

OCFbase = $328,900

NPVbase = –$780,000 + $328,900(PVIFA11%,4)

NPVbase = $240,394.39

The OCF and NPV for the worst case estimate are:

OCFworst = [($16,300 – 12,210)(162) – $588,500](.65) + .35($780,000 / 4)

OCFworst = $116,402

198 – SOLUTIONS

24. The marketing study and the research and development are both sunk costs and should be ignored.

The initial cost is the equipment plus the net working capital, so:

Initial cost = $29,400,000 + 3,500,000

Initial cost = $32,900,000

Next, we will calculate the sales and variable costs. Since we will lose sales of the expensive clubs

and gain sales of the cheap clubs, these must be accounted for as erosion. The total sales for the new

project will be:

Sales

New clubs

$825  74,000 = 61,050,000

Exp. clubs

$1,250  (–8,900) = –11,125,000

Cheap clubs

$375  11,000 = 4,125,000

$54,050,000

For the variable costs, we must include the units gained or lost from the existing clubs. Note that the

variable costs of the expensive clubs are an inflow. If we are not producing the sets anymore, we will

save these variable costs, which is an inflow. So:

Var. costs

New clubs

$370  74,000 = $27,380,000

CHAPTER 9 – 199

Change in NWC

–1,500,000

Total cash flow

–$20,000,000

Now we can begin the remaining calculations. Sales figures are given for each year, along with the

price per unit. The variable costs per unit are used to calculate total variable costs, and fixed costs are

given at $1,950,000 per year. To calculate depreciation each year, we use the initial equipment cost of

$18,500,000, times the appropriate MACRS depreciation each year. The remainder of each income

statement is calculated below. Notice at the bottom of the income statement we added back

depreciation to get the OCF for each year. The section labeled “Net cash flows” will be discussed

below:

200 – SOLUTIONS

Change in NWC

–873,300

–788,100

676,275

745,500

1,739,625

Capital spending

3,849,573

Total cash flow

$4,268,853

$6,347,003

$8,560,628

$7,274,228

$10,749,915

After we calculate the OCF for each year, we need to account for any other cash flows. The other cash

flows in this case are NWC cash flows and capital spending, which is the aftertax salvage of the

equipment. The required NWC is 15 percent of the change in sales in the next year. We will work

through the NWC cash flow for Year 1. The total NWC in Year 1 will be 15 percent of the sales

increase from Year 1 to Year 2, or:

Change in NWC for Year 1 = .15($23,962,500 – 29,784,500)

Change in NWC for Year 1 = –$873,300

Notice that the NWC cash flow is negative. Since the sales are increasing, we will have to spend more

money to increase NWC. In Year 3, the NWC cash flow is positive since sales are declining. And, in

Year 5, the NWC cash flow is the recovery of all NWC the company still has in the project.

CHAPTER 9 – 201

26. To find the initial pretax cost savings necessary to buy the new machine, we should use the tax shield

approach to find the OCF. We begin by calculating the depreciation each year using the MACRS

depreciation schedule. The depreciation each year is:

D1 = $535,000(.3333) = $178,315.50

D2 = $535,000(.4445) = $237,807.50

D3 = $535,000(.1481) = $79,233.50

D4 = $535,000(.0741) = $39,643.50

Using the tax shield approach, the OCF each year is:

OCF1 = (S – C)(1 – .35) + .35($178,315.50)

OCF2 = (S – C)(1 – .35) + .35($237,807.50)

OCF3 = (S – C)(1 – .35) + .35($79,233.50)

202 – SOLUTIONS

CHAPTER 10

SOME LESSONS FROM CAPITAL

MARKET HISTORY

Answers to Concepts Review and Critical Thinking Questions

9. The EMH only says, that within the bounds of increasingly strong assumptions about the information

10. a. If the market is not weak form efficient, then this information could be acted on and a profit

earned from following the price trend. Under (2), (3), and (4), this information is fully impounded

in the current price and no abnormal profit opportunity exists.

204 – SOLUTIONS

b. Under (2), if the market is not semi-strong form efficient, then this information could be used to

buy the stock “cheap” before the rest of the market discovers the financial statement anomaly.

Since (2) is stronger than (1), both imply that a profit opportunity exists; under (3) and (4), this

information is fully impounded in the current price and no profit opportunity exists.

c. Under (3), if the market is not strong form efficient, then this information could be used as a

1. The return of any asset is the increase in price, plus any dividends or cash flows, all divided by the

initial price. The return of this stock is:

R = [($85 – 72) + 1.65] / $72

R = .2035, or 20.35%

2. Using the equation for total return, we find:

R = [($62 – 72) + 1.65] / $72

R = –.1160, or –11.60%

And the dividend yield and capital gains yield are:

Dividend yield = $1.65 / $72

Dividend yield = .0229, or 2.29%

Capital gains yield = ($62 – 72) / $72

Capital gains yield = –.1389, or –13.89%

CHAPTER 10 – 205

4. The total dollar return is the change in price plus the coupon payment, so:

Total dollar return = $1,020 – 1,032 + 65

Total dollar return = $53

The nominal percentage return of the bond is:

206 – SOLUTIONS





539.15.21.08.36.

1









=

ii

Remembering back to “sadistics,” we calculate the variance of each stock as:

 

06203.146.39.146.15.146.21.146.08.146.36.

151

=−+−−+−+−−+−

−

=

Y



Total

19.41%

39.31%

–19.90%

a. The average return for large-company stocks over this period was:

Large-company stock average return = 19.41% / 6

Large-company stock average return = 3.24%

CHAPTER 10 – 207

And the average return for T-bills over this period was:

T-bills average return = 39.31% / 6

T-bills average return = 6.55%

b. Using the equation for variance, we find the variance for large-company stocks over this period

was:

Variance = 1/5[(–.1469 – .0324)2 + (–.2647 – .0324)2 + (.3723 – .0324)2 + (.2393 – .0324)2 +

(–.0716 – .0324)2 + (.0657 – .0324)2]

Variance = .058136

And the standard deviation for large company stocks over this period was:

Standard deviation = (.058136)1/2

Standard deviation = .2411, or 24.11%

Using the equation for variance, we find the variance for T-bills over this period was:

208 – SOLUTIONS

9. a. To find the average return, we sum all the returns and divide by the number of returns, so:

Arithmetic average return = (–.12 + .23 + .18 + .07 + .13) / 5

Arithmetic average return = .0980, or 9.80%

b. Using the equation to calculate variance, we find:

10. a. To calculate the average real return, we can use the average return of the asset, and the average

inflation rate in the Fisher equation. Doing so, we find:

(1 + R) = (1 + r)(1 + h)

r

= (1.0980 / 1.032) – 1

r

= .0640, or 6.40%

11. We can find the average real risk-free rate using the Fisher equation. The average real risk-free rate

was:

(1 + R) = (1 + r)(1 + h)

CHAPTER 10 – 209

13. To find the return on the zero-coupon bond, we first need to find the price of the bond today. We need

to remember that the price for zero-coupon bonds is calculated with semiannual periods. Since one

year has elapsed, the bond now has 19 years to maturity, so the price today is:

15. The return of any asset is the increase in price, plus any dividends or cash flows, all divided by the

initial price. This stock paid no dividend, so the return was:

APR = 25.40%

16. To find the real return each year, we will use the Fisher equation, which is:

1 + R = (1 + r)(1 + h)

Using this relationship for each year, we find:

T-bills

Inflation

Real Return

1926

.0330

–.0112

.0447

1927

.0315

–.0226

.0554

1928

.0405

–.0116

.0527

210 – SOLUTIONS

was 6.4 percent, with a standard deviation of 8.4 percent. The range of returns you would expect to

see 68 percent of the time is the mean plus or minus 1 standard deviation, or:

12.1 percent, with a standard deviation of 20.1 percent. The range of returns you would expect to see

68 percent of the time is the mean plus or minus 1 standard deviation, or:

19. Here, we know the average stock return, and four of the five returns used to compute the average

return. We can work the average return equation backward to find the missing return. The average

return is calculated as:

.10 = (.17 – .13 + .26 + .08 + R) / 5

CHAPTER 10 – 211

20. The arithmetic average return is the sum of the known returns divided by the number of returns, so:

Arithmetic average return = (–.23 + .09 + .37 –.08 + .28 +.19) / 6

Arithmetic average return = .1033, or 10.33%

21. To calculate the arithmetic and geometric average returns, we must first calculate the return for each

year. The return for each year is:

R1 = ($67.32 – 58.27 + 1.10) / $58.27 = .1742, or 17.42%

R2 = ($61.46 – 67.32 + 1.25) / $67.32 = –.0685, or –6.85%

22. To find the real return, we need to use the Fisher equation. Re-writing the Fisher equation to solve for

the real return, we get:

r = [(1 + R) / (1 + h)] – 1

212 – SOLUTIONS

So, the real return each year was:

Year

T-bill return

Inflation

Real return

1973

.0729

.0871

–.0131

1974

.0799

.1234

–.0387

1975

.0587

.0694

–.0100

1976

.0507

.0486

.0020

1977

.0545

.0670

–.0117

1978

.0764

.0902

–.0127

1979

.1056

.1329

–.0241

1980

.1210

.1252

–.0037

.6197

.7438

–.1120

a. The average return for T-bills over this period was:

CHAPTER 10 – 213

23. To find the return on the coupon bond, we first need to find the price of the bond today, so:

P1 = $65(PVIFA5.2%,9) + $1,000 / 1.0529

P1 = $1,091.58

was 6.1 percent, with a standard deviation of 10.0 percent. In the normal probability distribution,

approximately 2/3 of the observations are within one standard deviation of the mean. This means that

1/3 of the observations are outside one standard deviation away from the mean. Or:

Pr(R< –3.9 or R > 16.1)  1/3

But we are only interested in one tail here, that is, returns less than –3.9 percent, so:

Pr(R< –3.9)  1/6

You can use the z-statistic and the cumulative normal distribution table to find the answer as well.

Doing so, we find:

214 – SOLUTIONS

25. The mean return for small company stocks was 16.7 percent, with a standard deviation of 32.1 percent.

Doubling your money is a 100% return, so if the return distribution is normal, we can use the z-statistic.

So:

z = (X – µ) / 

27. Using the z-statistic, we find:

28. For each of the questions asked here, we need to use the z-statistic, which is:

z = (X – µ) / 

a. z1 = (10% – 6.4) / 8.4% = .4286

This z-statistic gives us the probability that the return is less than 10 percent, but we are looking

for the probability the return is greater than 10 percent. Given the symmetry of the normal

distribution, and the fact that the total probability is 100 percent (or 1), the probability of a return

greater than 10 percent is 1 minus the probability of a return less than 10 percent. Using the

NORMDIST function, we get:

Pr(R≥10%) = 1 – Pr(R≤10%) = 1 – .6659  33.41%

CHAPTER 10 – 215

For a return less than 0 percent:

CHAPTER 11

RISK AND RETURN

Answers to Concepts Review and Critical Thinking Questions

2. If the market expected the growth rate in the coming year to be 2 percent, then there would be no

3. a. systematic

CHAPTER 11 – 217

10. Earnings contain information about recent sales and costs. This information is useful for projecting

future growth rates and cash flows. Thus, unexpectedly low earnings often lead market participants to

reduce estimates of future growth rates and cash flows; lower prices are the result. The reverse is often

true for unexpectedly high earnings.

1. The portfolio weight of an asset is total investment in that asset divided by the total portfolio value.

First, we will find the portfolio value, which is:

2. The expected return of a portfolio is the sum of the weight of each asset times the expected return of

each asset. The total value of the portfolio is:

218 – SOLUTIONS

4. Here, we are given the expected return of the portfolio and the expected return of each asset in the

portfolio, and are asked to find the weight of each asset. We can use the equation for the expected

return of a portfolio to solve this problem. Since the total weight of a portfolio must equal 1 (100%),

the weight of Stock Y must be one minus the weight of Stock X. Mathematically speaking, this means:

E(Rp) = .122 = .13xX + .095(1 – xX)

7. The expected return of an asset is the sum of the probability of each state occurring times the

rate of return if that state occurs. So, the expected return of each asset is:

E(RA) = .10(.02) + .50(.10) + .40(.15)

E(RA) = .1120, or 11.20%

E(RB) = .10(–.30) + .50(.18) + .40(.31)

E(RB) = .1840, or 18.40%

CHAPTER 11 – 219

9. a. To find the expected return of the portfolio, we need to find the return of the portfolio in each

state of the economy. This portfolio is a special case since all three assets have the same weight.

To find the expected return in an equally weighted portfolio, we can sum the returns of each asset

and divide by the number of assets, so the expected return of the portfolio in each state of the

economy is:

Boom: Rp = (.15 + .02 + .34) / 3

Rp = .17, or 17%

Bust: Rp = (.03 + .16 − .08) / 3

Rp = .0367, or 3.67%

This is equivalent to multiplying the weight of each asset (1/3, or .3333) times its expected return

and summing the results, which gives:

Boom: Rp = 1/3(.15) + 1/3(.02) + 1/3(.34)

Rp = .17, or 17%

Bust: Rp = 1/3(.03) + 1/3(.16) + 1/3(–.08)

Rp = .0367, or 3.67%

220 – SOLUTIONS

10. a. This portfolio does not have an equal weight in each asset. We first need to find the return of the

portfolio in each state of the economy. To do this, we will multiply the return of each asset by its

portfolio weight and then sum the products to get the portfolio return in each state of the economy.

Doing so, we get:

Boom: Rp = .25(.35) + .50(.45) + .25(.33)

Rp = .3950, or 39.50%

Good: Rp = .25(.12) + .50(.10) + .25(.17)

Rp = .1225, or 12.25%

Poor: Rp = .25(.01) + .50(.02) + .25(–.05)

Rp = .0000, or 0.00%

Bust: Rp = .25(–.11) + .50(–.25) + .25(–.09)

CHAPTER 11 – 221

12. The beta of a portfolio is the sum of the weight of each asset times the beta of each asset. If the portfolio

is as risky as the market, it must have the same beta as the market. Since the beta of the market is one,

13. The CAPM states the relationship between the risk of an asset and its expected return. The CAPM is:

E(Ri) = Rf + [E(RM) – Rf] × i

222 – SOLUTIONS

14. We are given the values for the CAPM except for the  of the stock. We need to substitute these values

into the CAPM, and solve for the  of the stock. One important thing we need to realize is that we are

16. Here, we need to find the risk-free rate, using the CAPM. Substituting the values given, and solving

for the risk-free rate, we find:

17. a. Again, we have a special case where the portfolio is equally weighted, so we can sum the returns

of each asset and divide by the number of assets. The expected return of the portfolio is:

E(Rp) = (.117 + .035) / 2

E(Rp) = .0760, or 7.60%

b. We need to find the portfolio weights that result in a portfolio with a  of .7. We know the  of

the risk-free asset is zero. We also know the weight of the risk-free asset is one minus the weight

of the stock since the portfolio weights must sum to one, or 100 percent. So:

p = .70 = xS(1.23) + (1 – xS)(0)

.70 = 1.23xS + 0 – 0xS

xS = .70 / 1.23

xS = .5691

And, the weight of the risk-free asset is:

CHAPTER 11 – 223

xS = 2.46 / 1.23

xS = 2

18. First, we need to find the  of the portfolio. The  of the risk-free asset is zero, and the weight of the

risk-free asset is one minus the weight of the stock, so the  of the portfolio is:

ßp = xW(1.18) + (1 – xW)(0) = 1.18xW

So, to find the  of the portfolio for any weight of the stock, we simply multiply the weight of the

stock times its .

Even though we are solving for the  and expected return of a portfolio of one stock and the risk-free

asset for different portfolio weights, we are really solving for the SML. Any combination of this stock,

and the risk-free asset will fall on the SML. For that matter, a portfolio of any stock and the risk-free

224 – SOLUTIONS

19. There are two ways to correctly answer this question. We will work through both. First, we can use

the CAPM. Substituting in the value we are given for each stock, we find:

E(RY) = .041 + .07(1.20)

E(RY) = .1250, or 12.50%

It is given in the problem that the expected return of Stock Y is 11.6 percent, but according to the

CAPM, the return of the stock based on its level of risk, the expected return should be 12.5 percent.

This means the stock return is too low, given its level of risk. Stock Y plots below the SML and is

overvalued. In other words, its price must decrease to increase the expected return to 12.5 percent.

For Stock Z, we find:

E(RZ) = .041 + .07(.85)

E(RZ) = .1005, or 10.05%

The return given for Stock Z is 10.3 percent, but according to the CAPM the expected return of the

CHAPTER 11 – 225

20. We need to set the reward-to-risk ratios of the two assets equal to each other, which is:

(.116 – Rf) / 1.20 = (.103 – Rf) / .85

22. Here, we are given the expected return of the portfolio and the expected return of the assets in the

portfolio and are asked to calculate the dollar amount of each asset in the portfolio. So, we need to find

the weight of each asset in the portfolio. Since we know the total weight of the assets in the portfolio

must equal 1 (or 100%), we can find the weight of each asset as:

E(Rp) = .111 = .129xH + .0980(1 – xH)

xH = .4194

226 – SOLUTIONS

23. To find the expected return of the portfolio we first need to find the weight of each asset in the portfolio.

The weights of the assets sum to 1 (or 100%), so we can solve for the weights, using the betas of the

each asset and the beta of the portfolio. Doing so, we find:

24. To find the expected return of the portfolio, we first need to find the weight of each asset in the

portfolio. The weight of each asset is the dollar investment of that asset divided by the total dollar

value of the portfolio, so:

Portfolio value = 645($43) + 830($29) + 475($94) + 765($51)

Portfolio value = $135,470

And the weight of each asset in the portfolio is:

xW = 645($43) / $135,470

xW = .2047

CHAPTER 11 – 227

25. a. We need to find the return of the portfolio in each state of the economy. To do this, we will

multiply the return of each asset by its portfolio weight and then sum the products to get the

portfolio return in each state of the economy. Doing so, we get:

Boom: Rp = .40(.02) + .40(.32) + .20(.60)

Rp = .2560, or 25.60%

Normal: Rp = .40(.10) + .40(.12) + .20(.20)

Rp = .1280, or 12.80%

Bust: Rp = .40(.16) + .40(–.11) + .20(–.35)

Rp = –.0500, or –5.00%

And the expected return of the portfolio is:

26. We know that the reward-to-risk ratios for all assets must be equal. This can be expressed as:

[E(RA) – Rf] / A = [E(RB) – Rf] / ßB

The numerator of each equation is the risk premium of the asset, so:

228 – SOLUTIONS

27. We know the total portfolio value and the investment of two stocks in the portfolio, so we can find the

weight of these two stocks. The weights of Stock A and Stock B are:

xA = $105,000 / $500,000

xA = .21

xB = $155,000 / $500,000

xB = .31

Since the portfolio is as risky as the market, the  of the portfolio must be equal to one. We also know the

 of the risk-free asset is zero. We can use the equation for the  of a portfolio to find the weight of the

third stock. Doing so, we find:

28. We know the expected return of the portfolio and of each asset, but only one portfolio weight. We

need to recognize that the weight of the risk-free asset is one minus the weight of the other two assets.

Mathematically, the expected return of the portfolio is:

E(Rp) = .099 = .50(.128) + xF(.093) + (1 – .50 – xF)(.038)

CHAPTER 11 – 229

29. a. The expected return of an asset is the sum of the probability of each state occurring times the rate

of return if that state occurs. So, the expected return of each asset is:

E(RA) = .10(–.12) + .65(.09) + .25(.35) = .1340, or 13.40%

E(RB) = .10(–.05) + .65(.10) + .25(.21) = .1125, or 11.25%

b. We can use the expected returns we calculated to find the slope of the Security Market Line. We

know that the beta of Stock A is .25 greater than the beta of Stock B. Therefore, as beta increases

by .25, the expected return on a security increases by .0215 (= .1340 – .1125). The slope of the

security market line (SML) equals:

SlopeSML = Rise / Run

SlopeSML = Increase in expected return / Increase in beta

SlopeSML = (.1340 – .1125) / .25

SlopeSML = .0860, or 8.60%

230 – SOLUTIONS

30. The amount of systematic risk is measured by the  of an asset. Since we know the market risk

premium and the risk-free rate, if we know the expected return of the asset, we can use the CAPM to

solve for the  of the asset. The expected return of Stock I is:

E(RI) = .25(.02) + .60(.32) + .15(.18)

E(RI) = .2240, or 22.40%

Using the CAPM to find the  of Stock I, we find:

.2240 = .04 + .07I

I = 2.63

The total risk of the asset is measured by its standard deviation, so we need to calculate the standard

deviation of Stock I. Beginning with the calculation of the stock’s variance, we find:

I2 = .25(.02 – .2240)2 + .60(.32 – .2240)2 + .15(.18 – .2240)2

I2 = .01622

CHAPTER 12

COST OF CAPITAL

Answers to Concepts Review and Critical Thinking Questions

6. Two primary advantages of the SML approach are that the model explicitly incorporates the relevant

risk of the stock, and the method is more widely applicable than is the DGM model, since the SML

doesn’t make any assumptions about the firm’s dividends. The primary disadvantages of the SML

7. The appropriate aftertax cost of debt to the company is the interest rate it would have to pay if it were

to issue new debt today. Hence, if the YTM on outstanding bonds of the company is observed, the

232 – SOLUTIONS

8. a. This only considers the dividend yield component of the required return on equity.

10. If the different operating divisions were in much different risk classes, then separate cost of capital

figures should be used for the different divisions; the use of a single, overall cost of capital would be

inappropriate. If the single hurdle rate were used, riskier divisions would tend to receive funds for

investment projects, since their return would exceed the hurdle rate despite the fact that they may

actually plot below the SML and hence be unprofitable projects on a risk-adjusted basis. The typical

2. Here we have information to calculate the cost of equity, using the CAPM. The cost of equity is:

CHAPTER 12 – 233

3. We have the information available to calculate the cost of equity, using the CAPM and the dividend

growth model. Using the CAPM, we find:

RE = .035 + 1.10(.078)

RE = .1208, or 12.08%

And using the dividend growth model, the cost of equity is

4. To use the dividend growth model, we first need to find the growth rate in dividends. So, the increase

in dividends each year was:

g1 = ($1.33 – 1.24) / $1.24

g1 = .0726, or 7.26%

g2 = ($1.44 – 1.33) / $1.33

g2 = .0827, or 8.27%

$1.65 = $1.24(1 + g)4

g = .0740, or 7.40%

YTM = 5.60%

YTM = 5.74%

8. The book value of debt is the total par value of all outstanding debt, so:

CHAPTER 12 – 235

MVD = 1.07($145,000,000) + .674($75,000,000)

MVD = $205,700,000

YTM = 4.43%

So, the aftertax cost of the zero coupon bonds is:

9. a. Using the equation to calculate the WACC, we find:

WACC = .75(.1090) + .05(.051) + .20(.058)(1 – .35)

WACC = .0918, or 9.18%

236 – SOLUTIONS

11. Here, we have the WACC and need to find the debt–equity ratio of the company. Setting up the WACC

equation, we find:

WACC = .0865 = .104(E/V) + .053(D/V)(1 – .35)

Rearranging the equation, we find:

12. a. The book value of equity is the book value per share times the number of shares, and the book

value of debt is the face value of the company’s debt, so:

BVE = 3,900,000($11) = $42,900,000

BVD = $65,000,000 + 50,000,000 = $115,000,000

So, the total value of the company is:

V = $42,900,000 + 115,000,000 = $157,900,000

And the book value weights of equity and debt are:

E/V = $42,900,000 / $157,900,000 = .2717

D/V = 1 – E/V = .7283

b. The market value of equity is the share price times the number of shares, so:

CHAPTER 12 – 237

13. First, we will find the cost of equity for the company. The information provided allows us to solve for

the cost of equity using the dividend growth model, so:

YTM = 6.16%

To find the weighted average aftertax cost of debt, we need the weight of each bond as a percentage

of the total debt. We find:

xD1 = .98($65,000,000) / $112,200,000

xD1 = .5677

238 – SOLUTIONS

14. a. Using the equation to calculate WACC, we find:

15. We will begin by finding the market value of each type of financing. We find:

MVD = 15,500($1,000)(1.08) = $16,740,000

MVE = 495,000($81) = $40,095,000

MVP = 20,000($92) = $1,840,000

And the total market value of the firm is:

YTM = 5.79%

And the aftertax cost of debt is:

RD = (1 – .35)(.0579)

RD = .0376, or 3.76%

The cost of preferred stock is:

CHAPTER 12 – 239

16. a. We will begin by finding the market value of each type of financing. We find:

MVD = 125,000($1,000)(1.09) = $136,250,000

MVE = 5,400,000($64) = $345,600,000

MVP = 290,000($103) = $29,870,000

And the total market value of the firm is:

V = $136,250,000 + 345,600,000 + 29,870,000

V = $511,720,000

YTM = 5.93%

And the aftertax cost of debt is:

RD = (1 – .34)(.0593)

RD = .0391, or 3.91%

240 – SOLUTIONS

17. a. Projects Y and Z.

b. Using the CAPM to consider the projects, we need to calculate the expected return of the project,

given its level of risk. This expected return should then be compared to the expected return of the

18. We will begin by finding the market value of each type of financing. We find:

MVD = 13,000($1,000)(1.07) = $13,910,000

MVE = 345,000($73.50) = $25,357,500

MVP = 10,000($86) = $860,000

And the total market value of the firm is:

V = $13,910,000 + 25,357,500 + 860,000

V = $40,127,500

Now, we can find the cost of equity using the CAPM. The cost of equity is:

CHAPTER 12 – 241

YTM = 5.51%

And the aftertax cost of debt is:

RD = (1 – .35)(.0551)

RD = .0358, or 3.58%

19. The bonds have 26 years to maturity so the price today is:

P0 = $1,000 / (1 + .059 / 2)52

P0 = $220.51

The market value of the debt is:

20. To find the required return for the project, we need to adjust the company’s WACC for the level of

risk in the project. A debt–equity ratio of .48 implies a weight of debt of .48/1.48 and a weight of

equity of 1/1.48, so the company’s WACC is:

242 – SOLUTIONS

21. First, we need to find the project discount rate. The project discount rate is the company’s cost of

capital plus a risk adjustment factor. A debt–equity ratio of .25 implies a weight of debt of .25/1.25

and a weight of equity of 1/1.25, so the company’s WACC is:

WACC = (.25/1.25)(.032) + (1/1.25)(.104)

WACC = .0896, or 8.96%

Adjusting for risk, the project discount rate is:

MV4 = 1.057($85,000,000)

MV4 = $89,845,000

So, the total market value of the company’s debt is:

MVD = $31,500,000 + 47,700,000 + 67,470,000 + 89,845,000

MVD = $236,515,000

CHAPTER 12 – 243

The weight of each debt issue is:

YTM4 = 6.82%

The weighted average YTM of the company’s debt is thus:

244 – SOLUTIONS

23. a. Using the dividend discount model, the cost of equity is:

RE = [(1.05)(1.04) / $83] + .04

RE = .0532, or 5.32%

24. First, we need to find the adjusted cash flow from assets (ACFA) for each year. We are given the

projected EBIT, depreciation, increase in NWC, and capital spending. Each of these accounts increase

at 18 percent per year. So, the ACFA for each of the next five years will be:

Year 1

Year 2

Year 3

Year 4

Year 5

EBIT

$1,900,000

$2,242,000

$2,645,560

$3,121,761

$3,683,678

Depreciation

165,000

194,700

229,746

271,100

319,898

Taxes*

665,000

784,700

925,946

1,092,616

1,289,287

Capital

spending

115,000

135,700

160,126

188,949

222,959

Change in NWC

85,000

100,300

118,354

139,658

164,796

CHAPTER 12 – 245

25. The ACFA for each of the first five years will be the same as the previous problem. To calculate the

terminal value, we can use the price–sales ratio, which will be:

Terminal value5 = 3.5($9,500,000)

Terminal value5 = $33,250,000

The value of the company today is the present value of the first five FCFs, plus the value today of the

terminal value, or:

26. First, we need to find the adjusted cash flow from assets (ACFA) for each year. At the growth rates

given, the projected ACFA for each of the next five years will be:

Year 1

Year 2

Year 3

Year 4

Year 5

EBIT

$3,392,500

$3,901,375

$4,486,581

$5,159,568

$5,933,504

Depreciation

282,000

338,400

406,080

487,296

584,755

Taxes*

1,187,375

1,365,481

1,570,303

1,805,849

2,076,726

Capital spending

570,000

684,000

820,800

984,960

1,181,952

Change in NWC

115,500

127,050

139,755

153,731

169,104

ACFA

$1,801,625

$2,063,244

$2,361,803

$2,702,325

$3,090,477

246 – SOLUTIONS

27. We can use the debt–equity ratio to calculate the weights of equity and debt. The debt of the company

has a weight for long-term debt and a weight for accounts payable. We can use the weight given for

accounts payable to calculate the weight of accounts payable and the weight of long-term debt. The

weight of each will be:

Accounts payable weight = .15/1.15 = .1304

Long-term debt weight = 1/1.15 = .8696

Since the accounts payable has the same cost as the overall WACC, we can write the equation for the

WACC as:

28. a. The $4.5 million cost of the land 3 years ago is a sunk cost and irrelevant; the $5 million appraised

value of the land is an opportunity cost and is relevant. So, the total initial cash flow is:

CF0 = –$5,000,000 – 19,500,000 – 825,000

CF0 = –$25,325,000

CHAPTER 12 – 247

b. To find the required return for the project, we need to adjust the company’s WACC for the level

of risk in the project. We begin by calculating the market value of each type of financing, so:

MVD = 60,000($1,000)(.95) = $57,000,000

YTM = 6.61%

And the aftertax cost of debt is:

RD = (1 – .34)(.0661)

RD = .0436, or 4.36%

The cost of preferred stock is:

RP = $5.80 / $95

RP = .0611, or 6.11%

So, the company’s WACC is:

WACC = .0436($57,000,000 / $186,800,000) + .0611($8,550,000 / $186,800,000)

+ .1185($121,250,000 / $186,800,000)

WACC = .0930, or 9.30%

The company wants to use the subjective approach to this project because it is located overseas.

The adjustment factor is 2 percent, so the required return on this project is:

Project required return = .0930 + .02

Project required return = .1130, or 11.30%

248 – SOLUTIONS

c. The annual depreciation for the equipment will be:

$19,500,000 / 8 = $2,437,500

So, the book value of the equipment at the end of five years will be:

CHAPTER 13

LEVERAGE AND CAPITAL STRUCTURE

Answers to Concepts Review and Critical Thinking Questions

4. The more capital intensive industries, such as airlines, cable television, and electric utilities, tend to

250 – SOLUTIONS

9. One side is that Continental was going to go bankrupt because its costs made it uncompetitive. The

bankruptcy filing enabled Continental to restructure and keep flying. The other side is that Continental

10. As with any management decision, the goal is to maximize the value of shareholder equity. To

accomplish this with respect to the capital structure decision, management attempts to choose the

capital structure with the lowest cost of capital.

1. a. A table outlining the income statement for the three possible states of the economy is shown

below. The EPS is the net income divided by the 7,350 shares outstanding. The last row shows

%EPS

–35%

–

+20%

b. If the company undergoes the proposed recapitalization, it will repurchase:

Share price = Equity / Shares outstanding

Share price = $194,775 / 7,350

Share price = $26.50

Shares repurchased = Debt issued / Share price

Shares repurchased = $39,750 / $26.50

Shares repurchased = 1,500

2. a. A table outlining the income statement with taxes for the three possible states of the economy is

shown below. The share price is still $26.50, and there are still 7,350 shares outstanding. The last

%EPS

–35%

–

+20%

b. A table outlining the income statement with taxes for the three possible states of the economy

252 – SOLUTIONS

%ROE

–35%

–

+20%

The second row shows the percentage change in ROE from the normal economy.

4. a. Under Plan I, the unlevered company, net income is the same as EBIT with no corporate tax. The

EPS under this capitalization will be:

EPS = $600,000 / 300,000 shares

EPS = $2.00

CHAPTER 13 – 253

Under Plan II, the levered company, net income will be reduced by the interest payment. The

interest payment is the amount of debt times the interest rate, so:

NI = $600,000 – .10($2,367,000)

NI = $363,300

And the EPS will be:

EPS = $363,300 / 210,000 shares

EPS = $1.73

5. We can find the price per share by dividing the amount of debt used to repurchase shares by the number

of shares repurchased. Doing so, we find the share price is:

Share price = $2,367,000 / (300,000 – 210,000)

Share price = $26.30 per share

254 – SOLUTIONS

6. a. The income statement for each capitalization plan is:

EPS

$1.62

$2.63

$3.24

The all-equity plan has the highest EPS; Plan I has the lowest EPS.

b. The breakeven level of EBIT occurs when the capitalization plans result in the same EPS. The

EPS is calculated as:

EPS = (EBIT – RDD) / Shares outstanding

This equation calculates the interest payment (RDD) and subtracts it from the EBIT, which results

in the net income. Dividing by the shares outstanding gives us the EPS. For the all-equity capital

structure, the interest term is zero. To find the breakeven EBIT for two different capital structures,

we simply set the equations equal to each other and solve for EBIT. The breakeven EBIT between

the all-equity capital structure and Plan I is:

EBIT / 21,000 = [EBIT – .10($494,000)] / 11,500

EBIT = $109,200

And the breakeven EBIT between the all-equity capital structure and Plan II is:

CHAPTER 13 – 255

EPS

$1.05

$1.71

$2.10

The all-equity plan still has the highest EPS; Plan I still has the lowest EPS.

We can calculate the EPS as:

EPS = [(EBIT – RDD)(1 – TC)] / Shares outstanding

This is similar to the equation we used before, except now we need to account for taxes. Again,

the interest expense term is zero in the all-equity capital structure. So, the breakeven EBIT

between the all-equity plan and Plan I is:

7. To find the value per share of the stock under each capitalization plan, we can calculate the price as

the value of shares repurchased divided by the number of shares repurchased. So, under Plan I, the

value per share is:

256 – SOLUTIONS

8. a. The earnings per share are:

EPS = $19,300 / 6,400 shares

EPS = $3.02

Since all earnings are paid as dividends, the cash flow for the investors will be:

Cash flow = $3.02(100 shares)

Cash flow = $301.56

b. To determine the cash flow to the shareholder, we need to determine the EPS of the firm under

the proposed capital structure. The market value of the firm is:

V = $55(6,400)

V = $352,000

Under the proposed capital structure, the firm will raise new debt in the amount of:

D = .30($352,000)

D = $105,600

This means the number of shares repurchased will be:

Shares repurchased = $105,600 / $55

Shares repurchased = 1,920

Under the new capital structure, the company will have to make an interest payment on the new

debt. The net income with the interest payment will be:

NI = $19,300 – .08($105,600)

NI = $10,852

CHAPTER 13 – 257

9. a. The total value of the company is the share price times the number of shares, so:

V = $60(6,400)

V = $384,000

The investor will receive dividends in proportion to the percentage of the company’s shares they

own. The total dividends received by the shareholder will be:

Dividends received = $47,000($18,000 / $384,000)

Dividends received = $2,203.13

b. Under the proposed capital structure, the firm will raise new debt in the amount of:

D = .25($384,000)

D = $96,000

258 – SOLUTIONS

This means the number of shares repurchased will be:

Shares repurchased = $96,000 / $60

Shares repurchased = 1,600

Under the new capital structure, the company will have to make an interest payment on the new

debt. The net income with the interest payment will be:

NI = $47,000 – .07($96,000)

NI = $40,280

This means the EPS under the new capital structure will be:

EPS = $40,280 / (6,400 – 1,600)

EPS = $8.39

CHAPTER 13 – 259

10. a. With the information provided, we can use the equation for calculating WACC to find the cost

of equity. The equation for WACC (assuming no taxes) is:

WACC = (E/V)RE + (D/V)RD

The company has a debt–equity ratio of 1.5, which implies the weight of debt is 1.5/2.5, and the

weight of equity is 1/2.5, so:

WACC = .091 = (1/2.5)RE + (1.5/2.5)(.055)

RE = .1450, or 14.50%

11. a. For an all-equity financed company:

WACC = RE = .0960, or 9.60%

b. To find the cost of equity for the company with leverage, we need to use M&M Proposition I

with no taxes, so:

RE = RA + (RA – RD)(D/E)

RE = .0960 + (.0960 – .059)(.30/.70)

RE = .1119, or 11.19%

c. Using M&M Proposition I with no taxes again, we get:

260 – SOLUTIONS

12. Using M&M Proposition I with taxes, the value of the levered firm is:

13. The interest tax shield is the total interest paid times the tax rate, so:

14. M&M Proposition I with no taxes states the value of the levered firm is equal to the value of the

unlevered firm. So, with no taxes, the value of the firm if it issues debt is:

15. The value of the firm is the value of the debt plus the value of the equity. We can use this relationship

to find the value of equity in each case. So, the debt–equity ratio with no taxes is:

V = E + D

$33,600,000 = E + 19,400,000

E = $29,000,000

CHAPTER 13 – 261

So, the debt–equity ratio is:

16. When the company is all-equity financed, the cost of equity is:

WACC = RE = .0940, or 9.40%

Using M&M Proposition I with no taxes, the cost of equity will be:

17. a. With no debt, we are finding the value of an unlevered firm, so:

VU = EBIT(1 – TC) / RA

VU = $22,300(1 – .35) / .15

VU = $96,633.33

b. The general expression for the value of a leveraged firm is:

VL = VU + TCD

If debt is 50 percent of VU, then D = (.50)VU, and we have:

VL = VU + TC[(.50)VU]

VL = $96,633.33 + .35(.50)($96,633.33)

VL = $113,544.17

262 – SOLUTIONS

18. The return on equity is net income divided by equity. Net income can be expressed as:

NI = (EBIT – RDD)(1 – TC)

So, ROE is:

RE = (EBIT – RDD)(1 – TC)/E

Now we can rearrange and substitute as follows to arrive at M&M Proposition II with taxes:

RE = [EBIT(1 – TC)/E] – [RD(D/E)(1 – TC)]

RE = RAVU/E – [RD(D/E)(1 – TC)]

RE = RA(VL – TCD)/E – [RD(D/E)(1 – TC)]

RE = RA(E + D – TCD)/E – [RD(D/E)(1 – TC)]

RE = RA + (RA – RD)(D/E)(1 – TC)

And the equation for WACC is:

WACC = (E/V)RE + (D/V)RD(1 – TC)

Substituting the M&M Proposition II equation into the equation for WACC, we get:

WACC = (E/V)[RA + (RA – RD)(D/E)(1 – TC)] + (D/V)RD(1 – TC)

CHAPTER 13 – 263

Rearranging and reducing the equation, we get:

19. The return on equity is net income divided by equity. Net income can be expressed as:

NI = (EBIT – RDD)(1 – TC)

So, ROE is:

RE = (EBIT – RDD)(1 – TC)/E

Now we can rearrange and substitute as follows:

RE = [EBIT(1 – TC)/E] – [RD(D/E)(1 – TC)]

If we assume that EBIT and TC are constant, then we can treat the unlevered firm as a perpetuity whose

per-period cash flows are EBIT(1 – TC). Then:

VU = EBIT(1 – TC)/RA

264 – SOLUTIONS

20. M&M Proposition II, with no taxes is:

RE = RA + (RA – Rf)(D/E)

Note that we assumed the return on debt was the risk-free rate. This is an important assumption of

M&M Proposition II. The CAPM to calculate the cost of equity is expressed as:

RE = E(RM – Rf) + Rf

We can rewrite the CAPM to express the return on an unlevered company as:

RA = A(RM – Rf) + Rf

CHAPTER 14

DIVIDENDS AND DIVIDEND POLICY

Answers to Concepts Review and Critical Thinking Questions

3. First, relatively young and less profitable firms generally should not make cash distributions. They

need the cash to fund investments (and flotation costs discourage the raising of outside cash).

However, as a firm matures, it begins to generate free cash flow (which, you will recall, is internally

generated cash flow beyond that needed to fund profitable investment activities). Significant free cash

8. The plan will probably have little effect on shareholder wealth. The shareholders can reinvest on their

266 – SOLUTIONS

10. It would not be irrational to find low-dividend, high-growth stocks. The trust should be indifferent

between receiving dividends or capital gains since it does not pay taxes on either one (ignoring possible

restrictions on invasion of principal, etc.). It would be irrational, however, to hold municipal bonds.

1. With no taxes we would expect the stock price to drop by exactly the amount of the dividend, so the

new stock price will be:

2. Your total portfolio value will be the total stock value plus the dividends received, so:

3. The aftertax dividend is the pretax dividend times one minus the tax rate, so:

CHAPTER 14 – 267

4. a. The shares outstanding increases by 10 percent, so:

New shares outstanding = 35,000(1.10)

New shares outstanding = 38,500

New shares issued = 3,500

Since the par value of the new shares is $1, the capital surplus per share is $41. The additional

capital surplus is therefore:

Capital surplus on new shares = 3,500($41)

Capital surplus on new shares = $143,500

The new equity account balances will be:

Common stock ($1 par value)

$ 38,500

Capital surplus

292,500

5. a. To find the new shares outstanding, we multiply the current shares outstanding times the ratio of

old shares to new shares, so:

New shares outstanding = 35,000(2/1)

New shares outstanding = 70,000

The equity accounts are unchanged except that the par value of the stock is changed by the ratio

of new shares to old shares, so the new par value is:

268 – SOLUTIONS

6. To find the new stock price, we multiply the current stock price by the ratio of old shares to new

shares, so:

a. $86(3/5) = $51.60

b. $86(1/1.15) = $74.78

c. $86(1/1.425) = $60.35

7. The stock price is the total market value of equity divided by the shares outstanding, so:

$1.35 per share(23,000 shares) = $31,050

CHAPTER 14 – 269

8. Repurchasing the shares will reduce shareholders’ equity by $31,050. The shares repurchased will be

the total purchase amount divided by the stock price, so:

Shares bought = $31,050 / $31.50

Shares bought = 986

9. The stock price is the total market value of equity divided by the shares outstanding, so:

P0 = $650,000 equity / 24,000 shares

P0 = $27.08 per share

10. With a stock dividend, the shares outstanding will increase by one plus the dividend percentage, so:

New shares outstanding = 245,000(1.10)

New shares outstanding = 269,500

270 – SOLUTIONS

The capital surplus is the capital paid in excess of par value, which is $1, so:

Capital surplus for new shares = 24,500($52)

Capital surplus for new shares = $1,274,000

11. The only equity account that will be affected is the par value of the stock. The par value will change

by the ratio of old shares to new shares, so:

New par value = $1(1/2)

New par value = $.50 per share

The total dividends paid this year will be the dividend amount times the number of shares outstanding.

The company had 245,000 shares outstanding before the split. We must remember to adjust the shares

outstanding for the stock split, so:

12. a. If the company makes a dividend payment, we can calculate the wealth of a shareholder as:

Dividend per share = $9,065 / 4,900 shares

Dividend per share = $1.85

CHAPTER 14 – 271

The stock price after the dividend payment will be:

PE = 21.57

If the company repurchases stock, the number of shares will decrease. The total net income is the

PE = 21.57

13. a. The price of a share of stock is the present value of the dividends. The dividend per share each

year will be:

Year 1 dividend per share = $550,000 / 20,000 = $27.50

Year 2 dividend per share = $840,000 / 20,000 = $42

The required return is 12 percent, so the stock price today is:

P0 = $27.50 / 1.12 + $42 / 1.122 = $58.04

272 – SOLUTIONS

b. The dividend increase for existing shareholders for Year 1 will be the new dividend amount

minus by the current dividend amount, which is:

Dividend increase = $650,000 – 550,000 = $100,000

In order to pay this increase in dividends, the firm must sell new shares. The number of new

shares that must be sold is the dividend increase divided by the stock price, or:

14. Assuming no capital gains tax, the aftertax return for the Gordon Company is the capital gains growth

rate, plus the dividend yield times one minus the tax rate. Using the constant growth dividend model,

we get:

Aftertax return = g + D(1 – T) = .12

CHAPTER 14 – 273

15. Using the equation for the decline in the stock price ex-dividend for each of the tax rate policies,

we get:

(P0 – PX) / D = (1 – TP) / (1 – TG)

a. P0 – PX = D(1 – 0) / (1 – 0)

P0 – PX = D

b. P0 – PX = D(1 – .15) / (1 – 0)

P0 – PX = .85D

CHAPTER 15

RASING CAPITAL

Answers to Concepts Review and Critical Thinking Questions

10. a. The price will probably go up because IPOs are generally underpriced. This is especially true for

smaller issues, such as this one.

b. It is probably safe to assume that they are having trouble moving the issue, and it is likely that

the issue is not substantially underpriced.

1. If you receive 1,000 shares of each, the profit is:

2. Using X to stand for the required sale proceeds, the equation to calculate the total sale proceeds,

including flotation costs, is:

3. This is basically the same as the previous problem, except that we need to include the $1,425,000 of

expenses in the amount the company needs to raise, so:

4. We need to calculate the net amount raised and the costs associated with the offer. The net amount

raised is the number of shares offered times the price received by the company, minus the costs

associated with the offer, so:

Net amount raised = (9,500,000 shares)($22.32) – 1,475,000 – 350,000

Net amount raised = $210,215,000

276 – SOLUTIONS

The company received $210,215,000 from the stock offering. Now, we can calculate the direct costs.

Part of the direct costs are given in the problem, but the company also had to pay the underwriters.

The stock was offered at $24 per share, and the company received $22.32 per share. The difference,

which is the underwriters spread, is also a direct cost. The total direct costs were:

5. Using X to stand for the required sale proceeds, the equation to calculate the total sale proceeds,

including flotation costs, is:

6. This is basically the same as the previous problem, except that we need to include the $1,450,000 of

expenses in the amount the company needs to raise, so:

CHAPTER 15 – 277

7. We need to calculate the net amount raised and the costs associated with the offer. The net amount

raised is the number of shares offered times the price received by the company, minus the costs

associated with the offer, so:

Net amount raised = (7,750,000 shares)($31.75) – 1,350,000 – 210,000

Net amount raised = $244,502,500

The company received $244,502,500 from the stock offering. Now, we can calculate the direct costs.

Part of the direct costs are given in the problem, but the company also had to pay the underwriters.

The stock was offered at $34 per share, and the company received $31.75 per share. The difference,

which is the underwriters spread, is also a direct cost. The total direct costs were:

CHAPTER 16

SHORT-TERM FINANCIAL PLANNING

Answers to Concepts Review and Critical Thinking Questions

3. a. Use: The cash balance declined by $200 to pay the dividend.

10. The company will need less financing because it is essentially borrowing more from its suppliers.

Among other things, the company will likely need less short-term borrowing from other sources, so it

will save on interest expense.

CHAPTER 16 – 279

1. a. No change. A dividend paid for by the sale of debt will not change cash since the cash raised

from the debt offer goes immediately to shareholders.

b. No change. The real estate is paid for by the cash raised from the debt, so this will not change

the cash balance.

c. No change. Inventory and accounts payable will increase, but neither will impact the cash

account.

d. Decrease. The short-term bank loan is repaid with cash, which will reduce the cash balance.

e. Decrease. The payment of taxes is a cash transaction.

f. Decrease. The preferred stock will be repurchased with cash.

280 – SOLUTIONS

2. The total liabilities and equity of the company are the book value of equity, plus current liabilities and

long-term debt, so:

Total liabilities and equity = $13,465 + 1,630 + 8,200

Total liabilities and equity = $23,295

We have NWC other than cash. Since NWC is current assets minus current liabilities, NWC other than

cash is:

NWC other than cash = Accounts receivable + Inventory – Current liabilities

3. a. Increase. If receivables go up, the time to collect the receivables would increase, which increases

the operating cycle.

4. a. Increase; Increase. If the terms of the cash discount are made less favorable to customers, the

accounts receivable period will lengthen. This will increase both the cash cycle and the operating

cycle.

b. Increase; No change. This will shorten the accounts payable period, which will increase the cash

cycle. It will have no effect on the operating cycle since the length of the operating cycle is not

affected by the payables period.

c. Decrease; Decrease. If more customers pay in cash, the accounts receivable period will decrease.

This will decrease both the cash cycle and the operating cycle.

CHAPTER 16 – 281

5. a. 45-day collection period implies all receivables outstanding from previous quarter are collected

in the current quarter, and (90 – 45) / 90 = 1/2 of current sales are collected.

Q1

Q2

Q3

Q4

Beginning receivables

$330

$293

$338

$315

Sales

585

675

630

895

Cash collections

623

630

653

763

Ending receivables

$293

$338

$315

$448

b. 60-day collection period implies all receivables outstanding from previous quarter are collected

in the current quarter, and (90 – 60) / 90 = 1/3 of current sales are collected.

Q1

Q2

Q3

Q4

282 – SOLUTIONS

6. The operating cycle is the inventory period plus the receivables period. The inventory turnover and

inventory period are:

Inventory turnover = COGS / Average inventory

Inventory turnover = $77,681 / [($9,605 + 11,302) / 2]

Inventory turnover = 7.4311 times

Inventory period = 365 days / Inventory turnover

Inventory period = 365 days / 7.4311

Inventory period = 49.12 days

And the receivables turnover and receivables period are:

Receivables turnover = Credit sales / Average receivables

Receivables turnover = $124,652 / [($4,093 + 4,994) / 2]

Receivables turnover = 27.4352 times

Receivables period = 365 days / Receivables turnover

7. If we factor immediately, we receive cash on an average of 38 days sooner. The number of periods in

a year is:

CHAPTER 16 – 283

8. a. The payables period is zero since the company pays immediately. The payment in each period is

30 percent of next period’s sales, so:

Q1 Q2 Q3 Q4

Payment of accounts $229.50 $274.50 $252.00 $234.60

10. a. The November sales must have been the total uncollected sales minus the uncollected sales from

December, divided by the collection rate two months after the sale, so:

November sales = ($97,000 – 71,000) / .15

November sales = $173,333.33

284 – SOLUTIONS

b. The December sales are the uncollected sales from December divided by the sum of the collection

rates from the previous two months’ sales, so:

11. The sales collections each month will be:

Sales collections = .35(Current month sales) + .60(Previous month sales)

Given this collection, the cash budget will be:

April

May

June

Beginning cash balance

$152,000

$221,100

$361,850

12. a. 45-day collection period implies all receivables outstanding from previous quarter are collected

in the current quarter, and (90 – 45) / 90 = 1/2 of current sales are collected.

Q1

Q2

Q3

Q4

Beginning receivables

$1,900

$2,150

$2,600

$2,400

Sales

4,300

5,200

4,800

4,000

Cash collections

4,050

4,750

5,000

4,400

Ending receivables

$2,150

$2,600

$2,400

$2,000

CHAPTER 16 – 285

b. 60-day collection period implies all receivables outstanding from previous quarter are collected

in the current quarter, and (90 – 60) / 90 = 1/3 of current sales are collected.

13. a. The EAR of the loan without the compensating balance is:

EAR = (1 + .00485)12 – 1

EAR = .0598, or 5.98%

However, with the compensating balance, you will only get the use of part of the amount, so the

EAR with the compensating balance will be:

286 – SOLUTIONS

EAR = 1.00494 – 1

EAR = .0197, or 1.97%

b. To calculate the EAR of the loan, we can divide the interest on the loan by the amount of the

loan. The interest on the loan includes the opportunity cost of the compensating balance. The

opportunity cost is the amount of the compensating balance times the potential interest rate you

could have earned. The compensating balance is only on the unused portion of the credit line, so:

15.94 = $445,000 / Average payables

Average payables = $27,919.18

16. Since the company has a 32-day collection period, only those sales made in the first 58 days of the

quarter will be collected in that quarter. Total cash collections in the first quarter will be:

Q1 cash collections = Beginning receivables + (58/90)(Quarter 1 sales)

Q1 cash collections = $218 + (58/90)($378)

Q1 cash collections = $462

And cash collection in the second quarter will be sales made in the first 58 days of the quarter plus

sales made in the last 32 days of the first quarter, so:

Q2 cash collections = (58/90)(Quarter 2 sales) + (32/90)(Quarter 1 sales)

Q2 cash collections = (58/90)($468) + (32/90)($378)

Q2 cash collections = $436

Quarter 3 and Quarter 4 collections will be:

Q3 cash collections = (58/90)(Quarter 3 sales) + (32/90)(Quarter 2 sales)

Q3 cash collections = (58/90)($570) + (32/90)($468)

Q3 cash collections = $534

Q4 cash collections = (58/90)(Quarter 4 sales) + (32/90)(Quarter 3 sales)

Q4 cash collections = (58/90)($522) + (32/90)($570)

Q4 cash collections = $539

288 – SOLUTIONS

Minimum cash balance

–20

17. a. For every dollar borrowed, you pay quarterly interest of:

Interest = $1(.0173) = $.0173

You also must maintain a compensating balance of 5 percent of the funds borrowed, so for each

dollar borrowed, you will only receive:

Amount received = $1(1 – .05) = $.95

We can adjust the EAR equation we have been using to account for the compensating balance by

dividing the EAR by one minus the compensating balance, so:

EAR = [(1.0173)4 – 1] / (1 – .05)

EAR = .0748, or 7.48%

$1.02102 = $.95(1 + R)

R = $1.02102 / $.95 – 1

EAR = .0748, or 7.48%

b. The EAR is the amount of interest paid on the loan divided by the amount received when the loan

is originated. The amount of interest you will pay on the loan is the amount of the loan times the

effective annual interest rate, so:

Interest = $210,000,000[(1.0173)4 – 1]

Interest = $14,913,473.49

For whatever loan amount you take, you will only receive 95 percent of that amount since you

must maintain a 5 percent compensating balance on the portion of the credit line used. The credit

line also has a fee of .25 percent, so you will only get to use:

290 – SOLUTIONS

18. You will pay interest of:

Interest = $20,000,000(.065) = $1,300,000

Additionally, the compensating balance on the loan is:

Compensating balance = $20,000,000(.04) = $800,000

Since this is a discount loan, you will receive the loan amount minus the interest payment. You will

also not get to use the compensating balance. So, the amount of money you will actually receive on a

$20 million loan is:

Cash received = $20,000,000 – 1,300,000 – 800,000

Cash received = $17,900,000

CHAPTER 17

WORKING CAPITAL MANAGEMENT

Answers to Concepts Review and Critical Thinking Questions

8. a. About the only disadvantage to holding T-bills are the generally lower yields compared to

alternative money market investments.

292 – SOLUTIONS

13. a. A sight draft is a commercial draft that is payable immediately.

7. Customer type

CHAPTER 17 – 293

18. a. B: A is likely to sell for cash only, unless the product really works. If it does, then they might

grant longer credit periods to entice buyers.

19. The three main categories of inventory are: raw material (initial inputs to the firm’s production

20. JIT systems reduce inventory amounts. Assuming no adverse effects on sales, inventory turnover will

increase. Since assets will decrease, total asset turnover will also increase. Recalling the Du Pont

equation, an increase in total asset turnover, all else being equal, has a positive effect on ROE.

2. The available balance is the amount you have on deposit, or $12,700. This is a collection float since

294 – SOLUTIONS

4. a. There are 30 days until account is overdue. If you take the full period, you must remit:

Remittance = 540($62)

Remittance = $33,480

b. There is a 1 percent discount offered, with a 10 day discount period. If you take the discount, you

will only have to remit:

5. The average daily receipts are the total amount of checks received divided by the number of days in a

month. Assuming 30 days in a month, the average daily float is:

6. a. The disbursement float is the average monthly checks written times the average number of days

for the checks to clear, so:

Disbursement float = 4($23,400)

Disbursement float = $93,600

CHAPTER 17 – 295

The collection float is the average monthly checks received times the average number of days for

the checks to clear, so:

7. The total sales of the firm are equal to the total credit sales since all sales are on credit, so:

Total credit sales = 7,400($235)

Total credit sales = $1,739,000

The average collection period is the percentage of accounts taking the discount times the discount

period, plus the percentage of accounts not taking the discount times the days’ until full payment is

required, so:

Average collection period = .40(10) + .60(30)

Average collection period = 22 days

296 – SOLUTIONS

8. The receivables turnover is 365 divided by the average collection period, so:

9. a. The average collection period is the percentage of accounts taking the discount times the discount

period, plus the percentage of accounts not taking the discount times the days’ until full payment

is required, so:

10. The daily sales are:

11. The interest rate for the term of the discount is:

30 – 10 = 20 days

So, using the EAR equation, the effective annual interest rate is:

EAR = (1 + Periodic rate)m – 1

EAR = (1.0101)365/20 – 1

EAR = .2013, or 20.13%

CHAPTER 17 – 297

a. The periodic interest rate is:

12. The receivables turnover is:

Receivables turnover = 365 / Average collection period

13. The carrying costs are the average inventory times the cost of carrying an individual unit, so:

Carrying costs = (1,150 / 2)($6.75)

Carrying costs = $3,881.25

The order costs are the number of orders times the cost of an order, so:

Restocking costs = 52($425)

Restocking costs = $22,100

The economic order quantity is:

298 – SOLUTIONS

14. The carrying costs are the average inventory times the cost of carrying an individual unit, so:

Carrying costs = (765 / 2)($27)

Carrying costs = $10,327.50

EOQ = 511.66

The number of orders per year will be the total units sold per year divided by the EOQ, so:

15. The total carrying costs are:

Carrying costs = (Q/2)  CC

where CC is the carrying cost per unit. The restocking costs are:

Restocking costs = F  (T/Q)

CHAPTER 17 – 299

Q–2 = CC/ (2  F  T)

Q2 = (2  F  T) / CC

Q = [(2  F  T) / CC]1/2

16. Since the company sells 700 suits per week, and there are 52 weeks per year, the total number of suits

sold is:

Total suits sold = 700 × 52 = 36,400

And, the EOQ is 500 suits, so the number of orders per year is:

Orders per year = 36,400 / 500 = 72.80

CHAPTER 18

INTERNATIONAL ASPECTS OF

FINANCIAL MANAGEMENT

Answers to Concepts Review and Critical Thinking Questions

CHAPTER 18 – 301

8. a. False. If prices are rising faster in Great Britain, it will take more pounds to buy the same amount

of goods that one dollar can buy; the pound will depreciate relative to the dollar.

9. a. American exporters: their situation in general improves because a sale of the exported goods for

a fixed number of pesos will be worth more dollars.

American importers: their situation in general worsens because the purchase of the imported

goods for a fixed number of pesos will cost more in dollars.

10. False. If the financial markets are perfectly competitive, the difference between the Eurodollar rate

and the U.S. rate will be due to differences in risk and government regulation. Therefore, speculating

in those markets will not be beneficial.

b. $1.2452

302 – SOLUTIONS

c. €5,000,000($1.2452/€) = $6,226,000

Alternatively the question can be answered as:

€5,000,000 / (€.8031/$) = $6,225,875

(£100)($1.5649/£1)(C$1.1417/$1) = C$178.66

3. a. F180 = ¥118.37(per $). The yen is selling at a premium because it is more expensive in the forward

market than in the spot market ($.008430 versus $.008448).

4. a. The U.S. dollar, since one Canadian dollar will buy:

(Can$1)/(Can$1.09/$1) = $.9174

CHAPTER 18 – 303

b. The cost in U.S. dollars is:

(Can$2.49)/(Can$1.09/$1) = $2.28

5. a. The cross rate in ¥/£ terms is:

(¥121/$1)($1.53/£1) = ¥185.13/£1

6. We can rearrange the approximate interest rate parity condition to answer this question. The equation

we will use is:

RFC = (Ft – S0) / S0 + RUS

304 – SOLUTIONS

7. If we invest in the U.S. for the next three months, we will have:

$30,000,000(1.0019)3 = $30,171,325.11

9. The profit will be the quantity sold, times the sales price minus the cost of production. The production

cost is in Singapore dollars, so we must convert this to U.S. dollars. Doing so, we find that if the

exchange rate stays the same, the profit will be:

Profit = 30,000[$150 – {(S$185.50)/(S$1.3043/$1)}]

Profit = $233,343.56

If the exchange rate rises, we must adjust the cost by the increased exchange rate, so:

Profit = 30,000[$150 – {(S$185.50)/1.1(S$1.3043/$1)}]

Profit = $621,221.41

CHAPTER 18 – 305

10. a. If IRP holds, then:

F180 = (W1,108.27)[1 + (.031 – .025)]1/2

F180 = W1,111.5898

$1.01330 – 1.01225 = $.00104

11. a. The yen is expected to get stronger, since it will take fewer yen to buy one dollar in the future

than it does today.

12. We need to find the change in the exchange rate over time, so we need to use the interest rate parity

relationship:

Ft = S0 × [1 + (RFC – RUS)]t

Using this relationship, we find the exchange rate in one year should be:

306 – SOLUTIONS

13. Pounds are cheaper in New York, so we start there. Buy:

$10,000(£/$1.5769) = £6,341.56

15. a. To construct the balance sheet in dollars, we need to convert the account balances to dollars. At

the current exchange rate, we get:

Assets = solaris 40,000  ($/solaris 1.20) = $33,333.33

Debt = solaris 12,500  ($/solaris 1.20) = $10,416.67

Equity = solaris 27,500  ($/solaris 1.20) = $22,916.67

b. In one year, if the exchange rate is solaris 1.30/$, the accounts will be:

CHAPTER 18 – 307

16. First, we need to construct the end of year balance sheet in solaris. Since the company has retained

earnings, the equity account will increase, which necessarily implies the assets will also increase by

the same amount. So, the balance sheet at the end of the year in solaris will be:

Balance Sheet (solaris)